Prof. Chenfeng Li FLSW
| Director, Zienkiewicz Institute for Modelling, Data and AI | Post: Faculty of Science and Engineering, Swansea University Bay Campus, Swansea SA1 8EN, UK. |
| Non-executive Director, Temporary Works Forum | Tel: +44-01792-602256 |
| Editor-in-Chief, Engineering Computations | Email: c.f.li |
SPECIALIST SUBJECTS
RESEARCH INTERESTS
|
|
|
|
JOURNAL Papers
2023
 |
 |
|
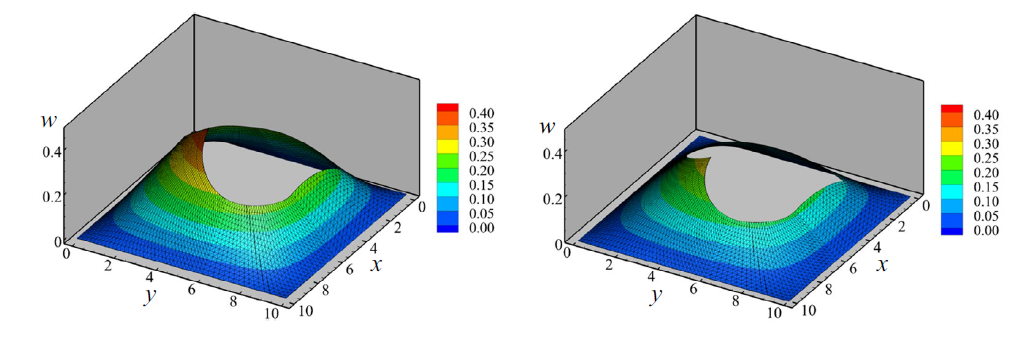 |
||
 |
 |

 |
 |
|
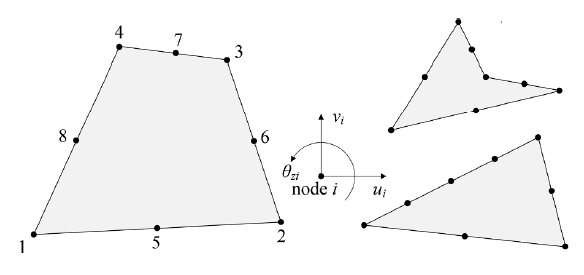 |
||
 |
 |

 |
 |
|
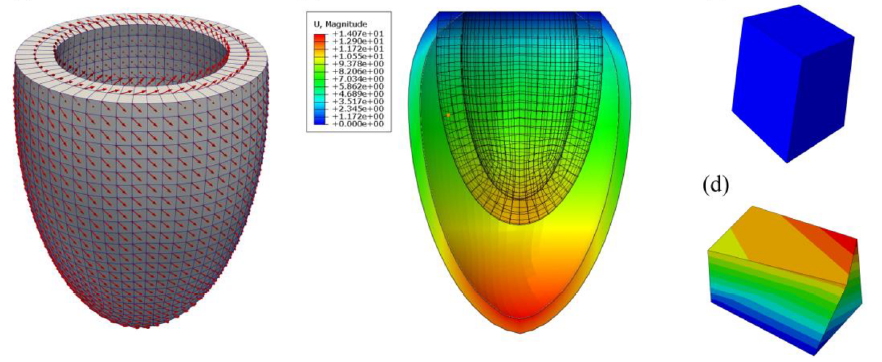 |
||
 |
 |

 |
 |
|
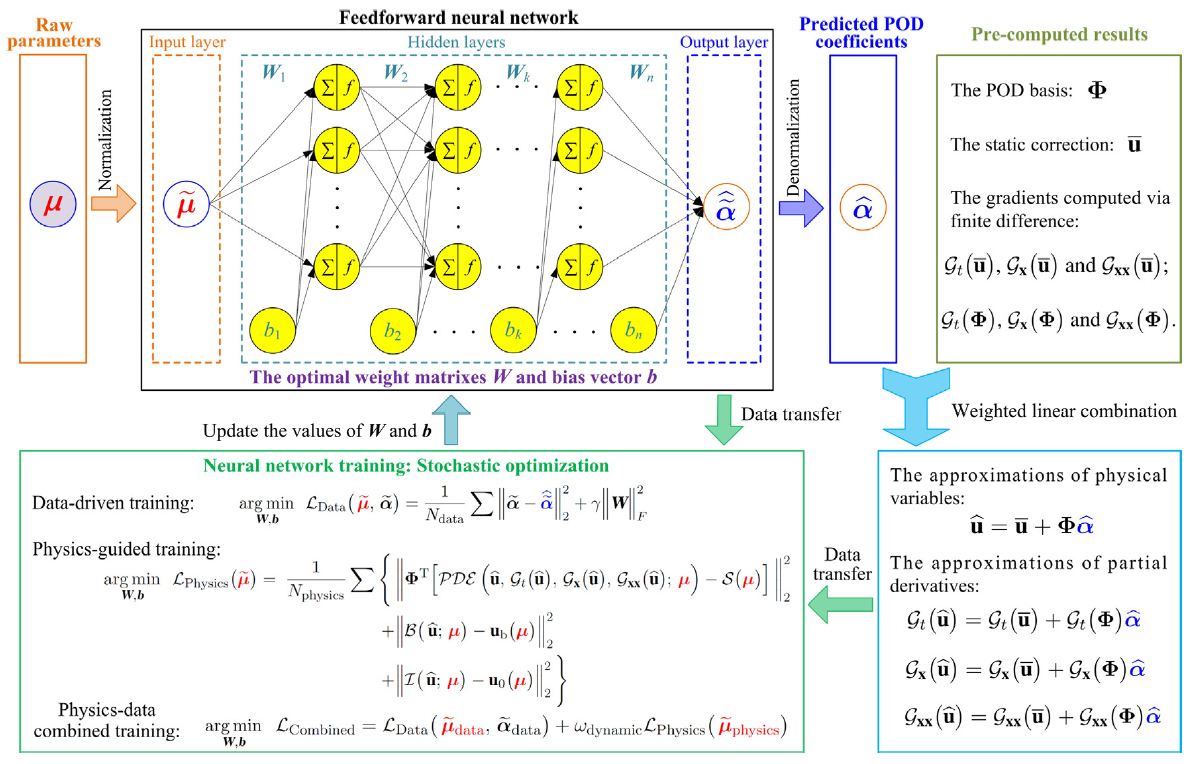 |
||
 |
 |

2022
 |
 |
|
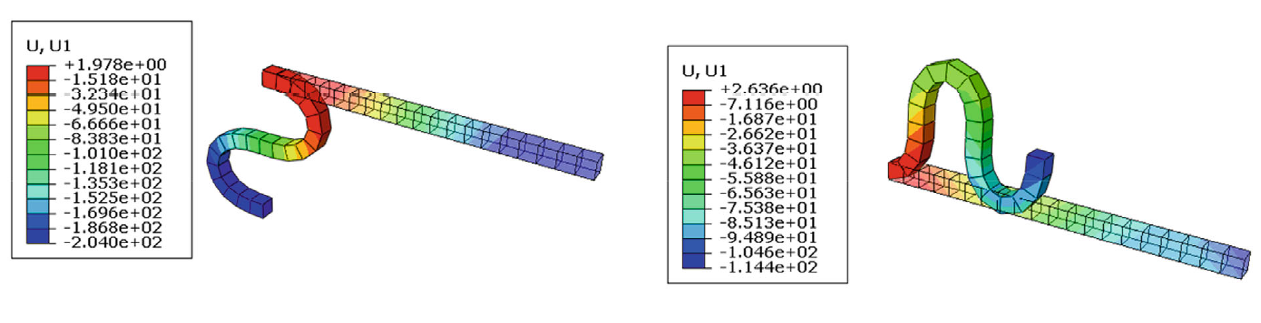 |
||
 |
 |

 |
 |
|
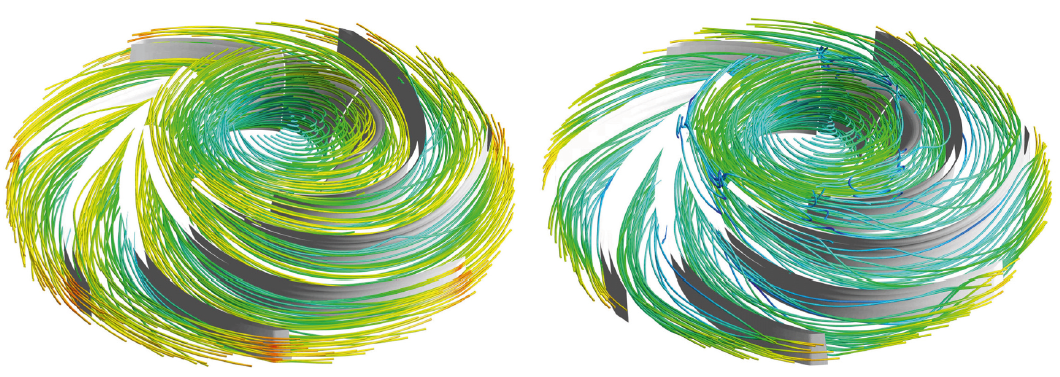 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
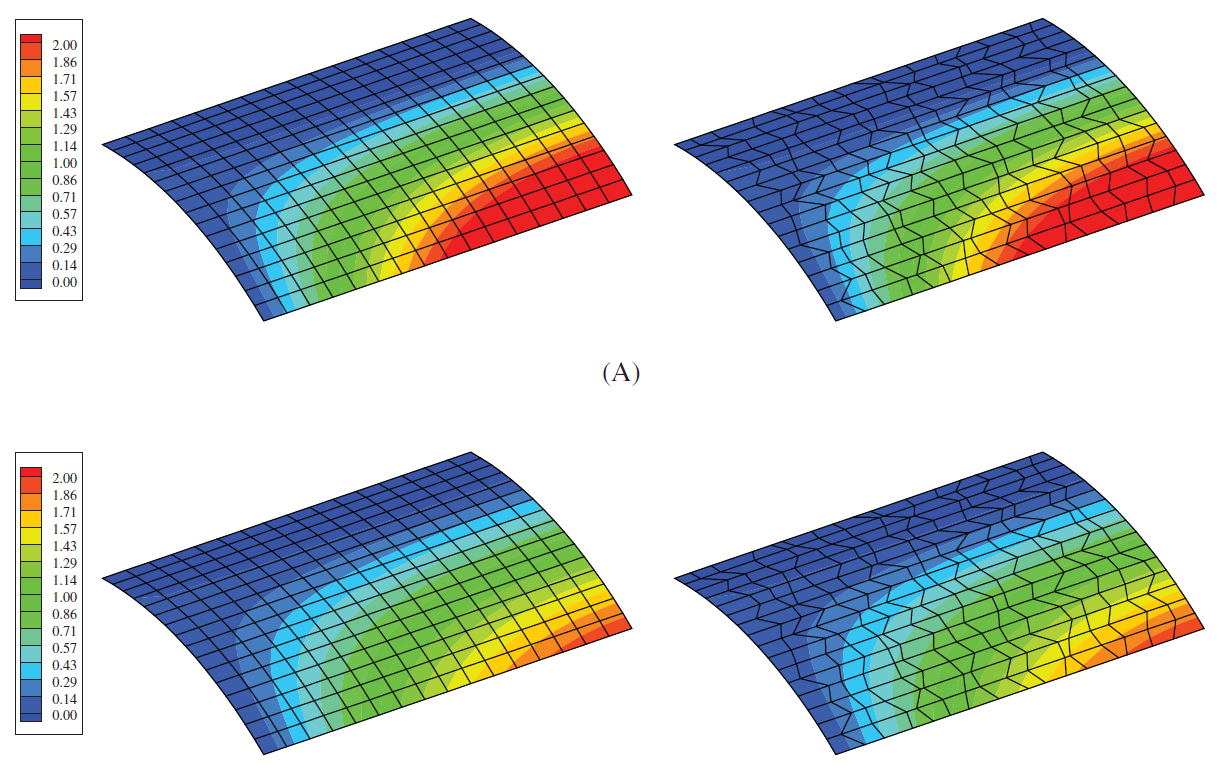 |
||
 |
 |

 |
 |
|
 |
||
 |
 |


 |
 |
|
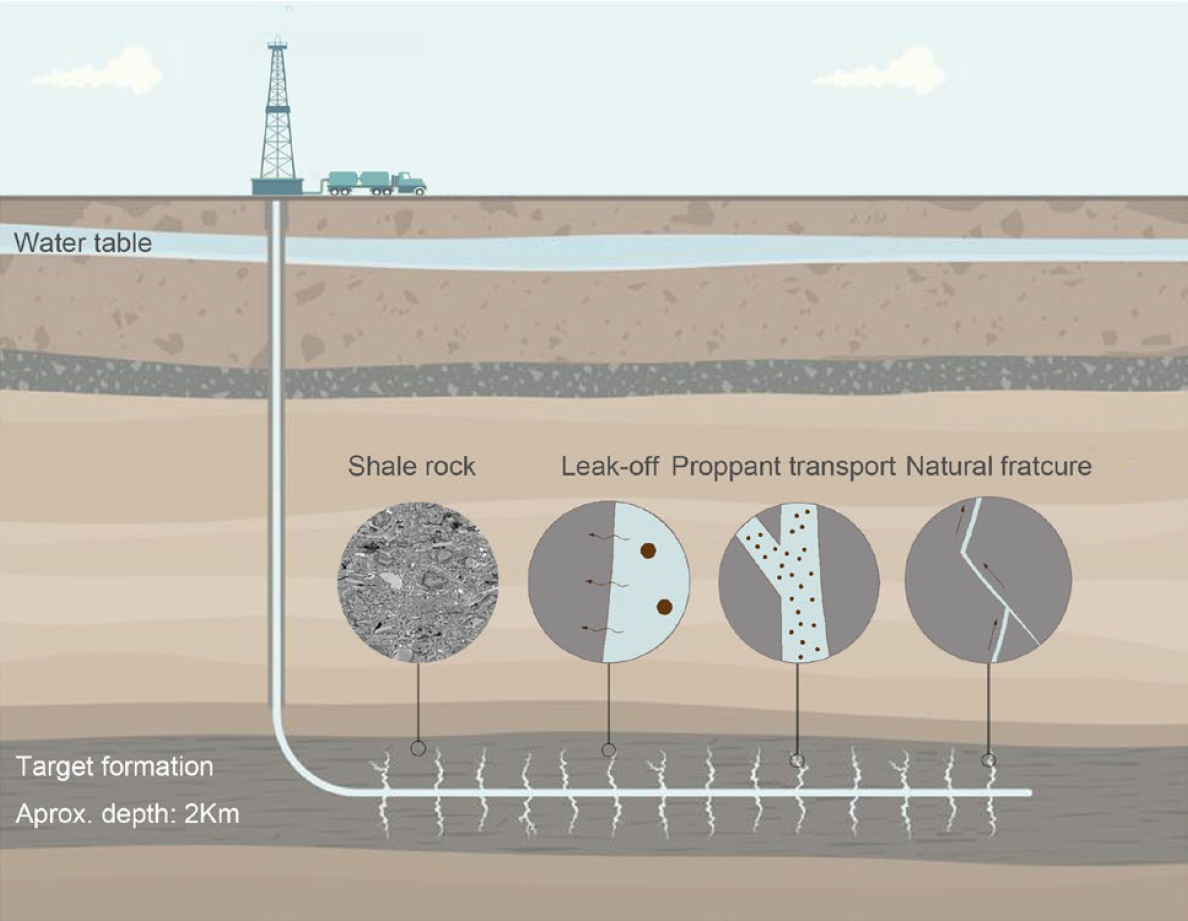 |
||
 |
 |

 |
 |
|
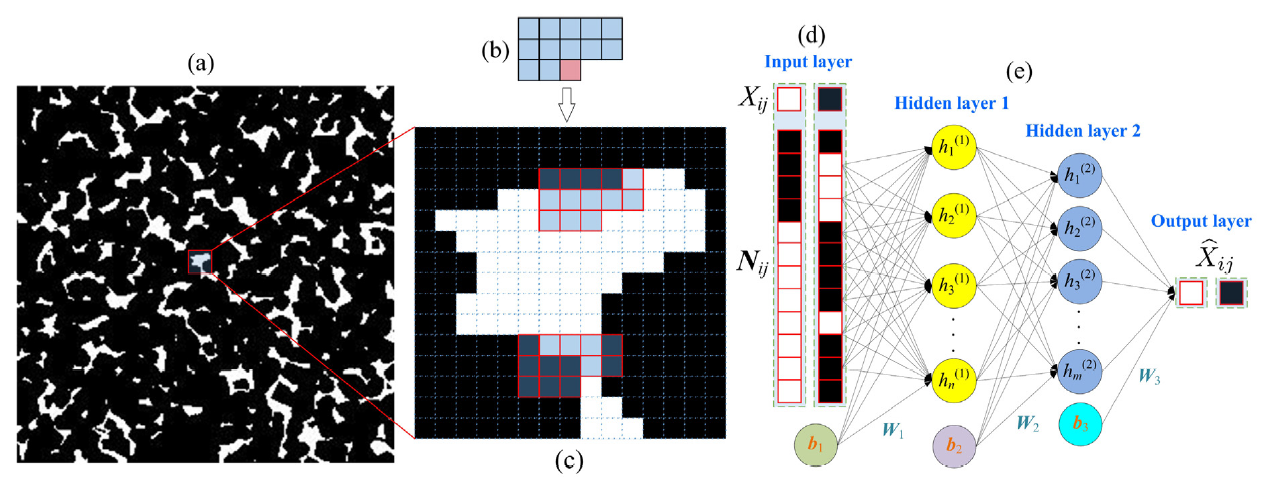 |
||
 |
 |

 |
 |
|
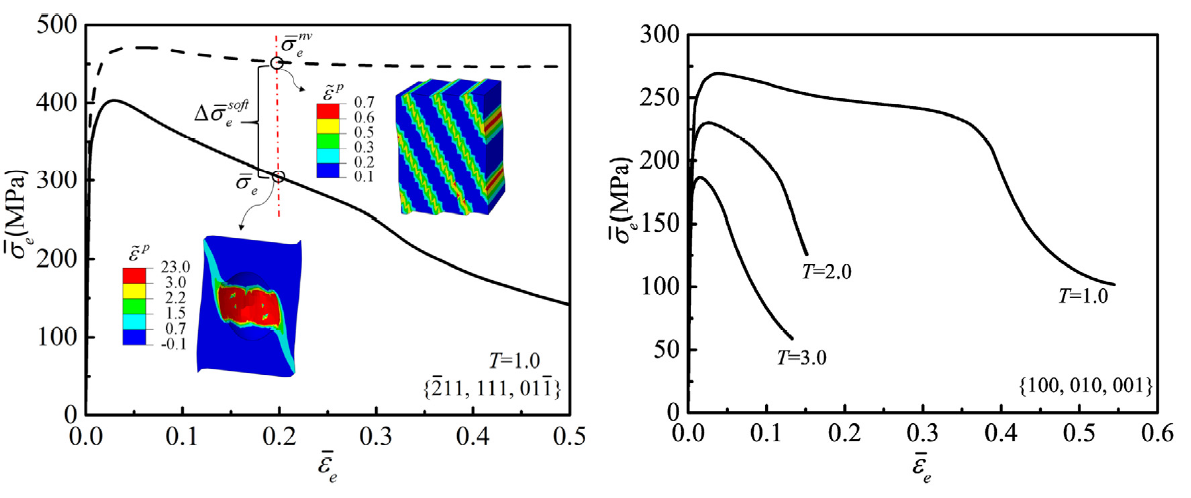 |
||
 |
 |

 |
 |
|
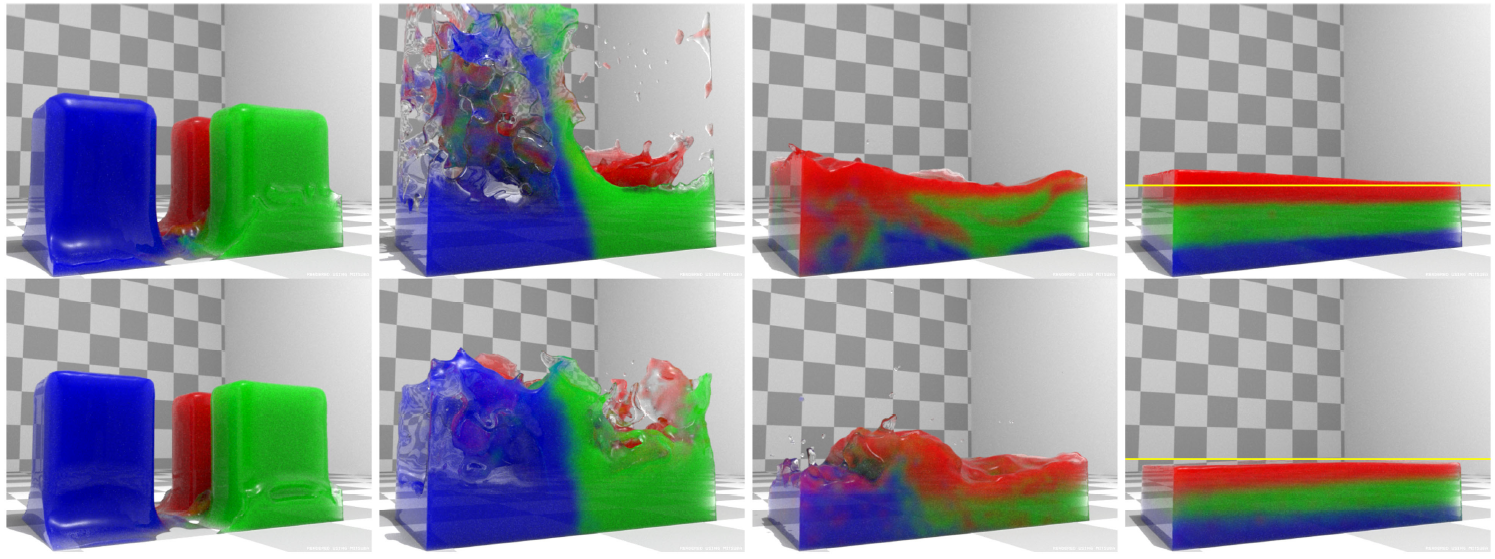 |
||
 |
 |


2021
 |
 |
|
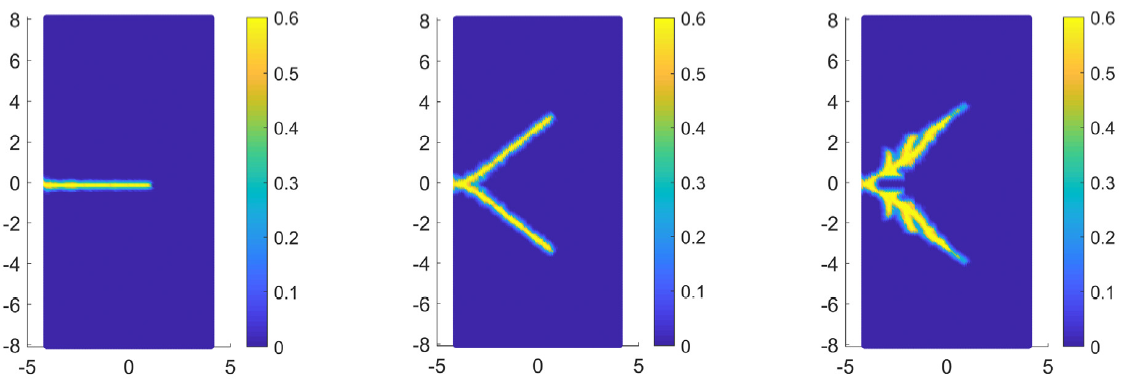 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
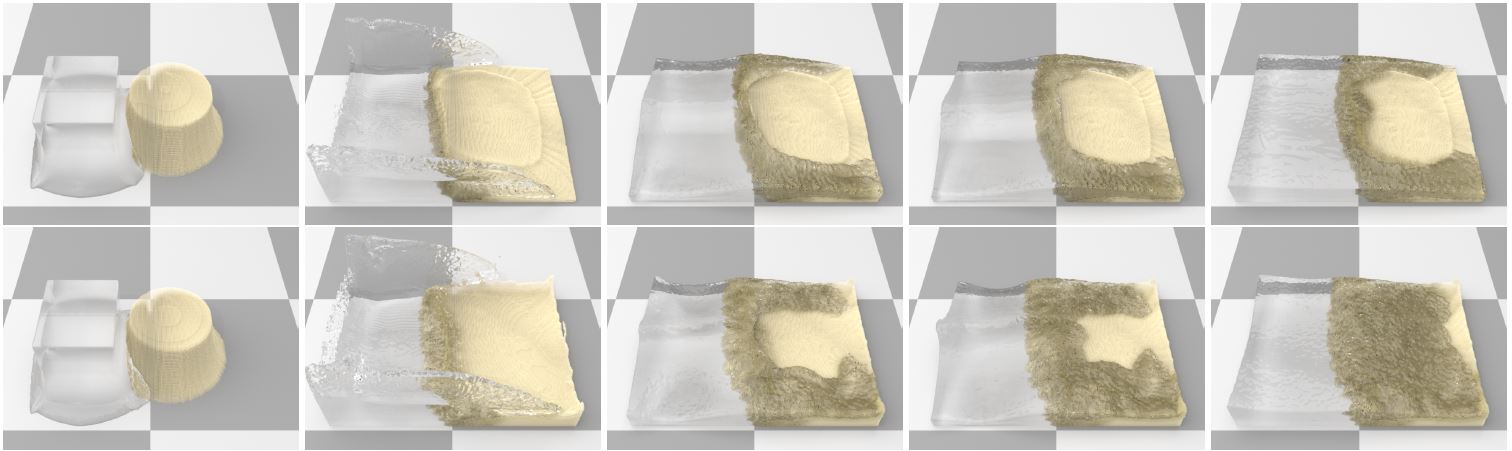 |
||
 |
 |


 |
 |
|
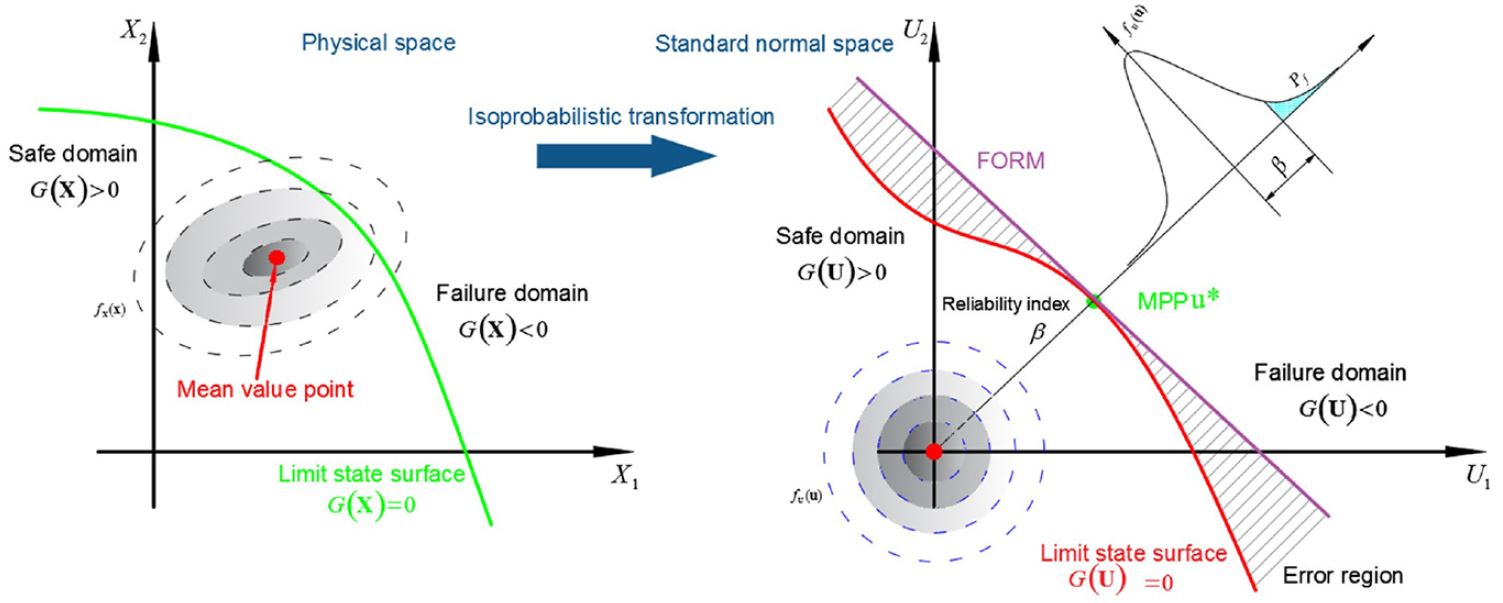 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
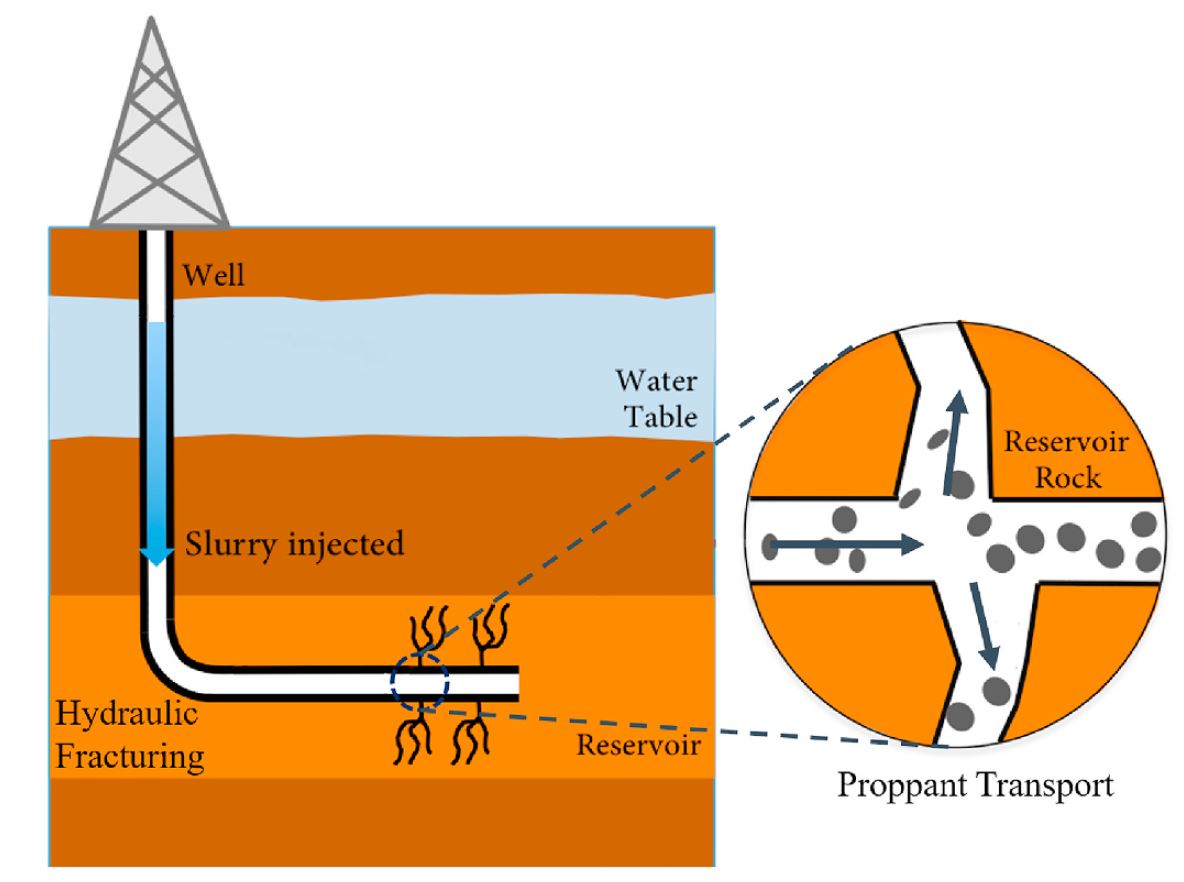 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
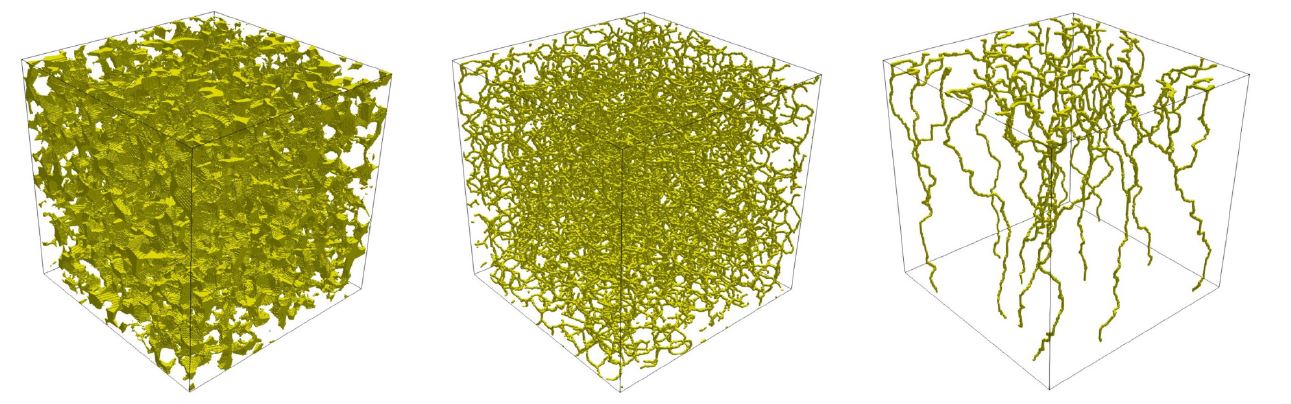 |
||
 |
 |

 |
 |
|
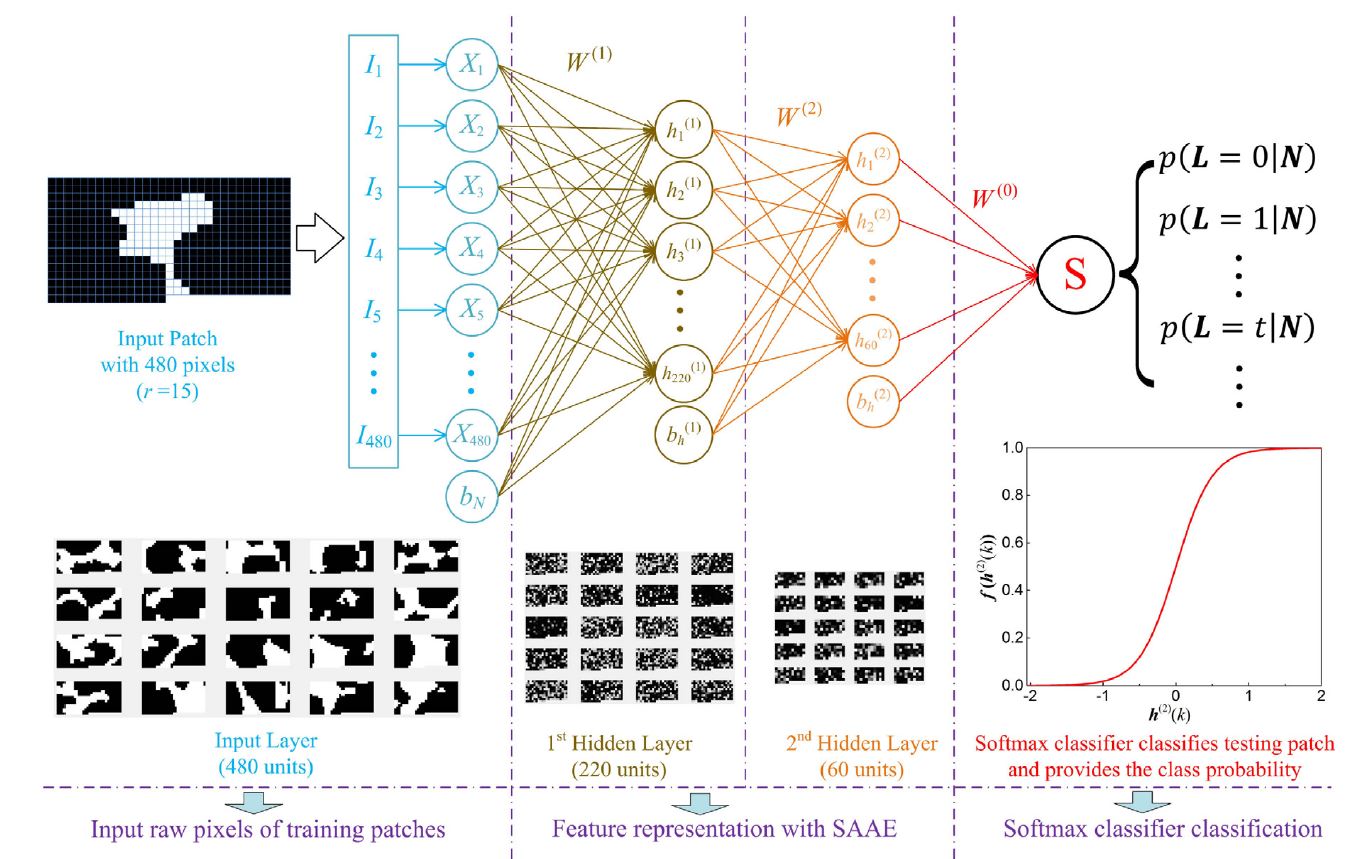 |
||
 |
 |

2020
 |
 |
|
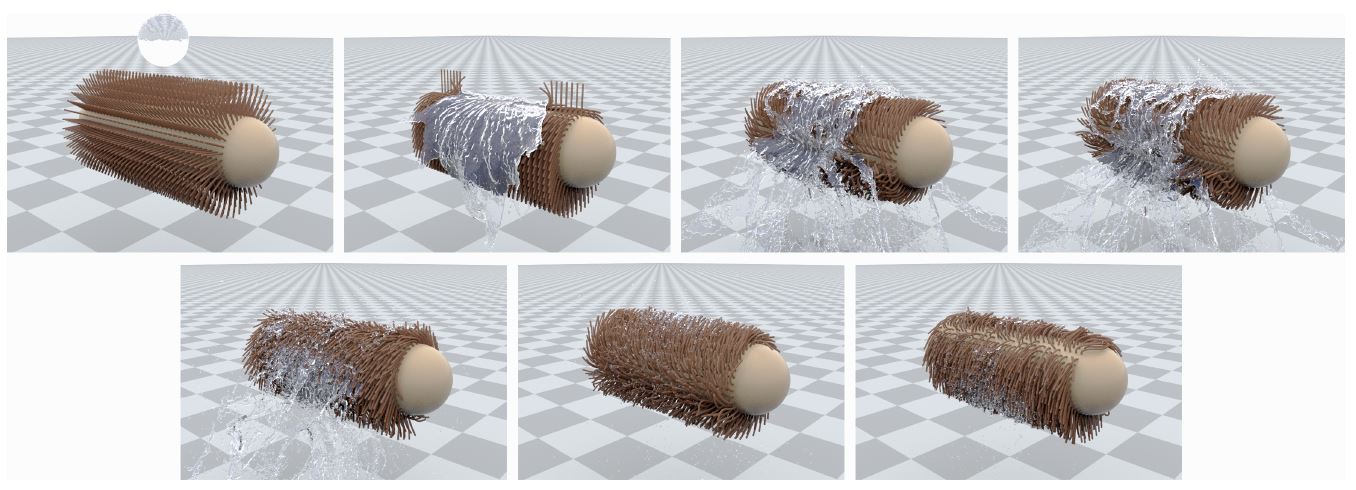 |
||
 |
 |


 |
 |
|
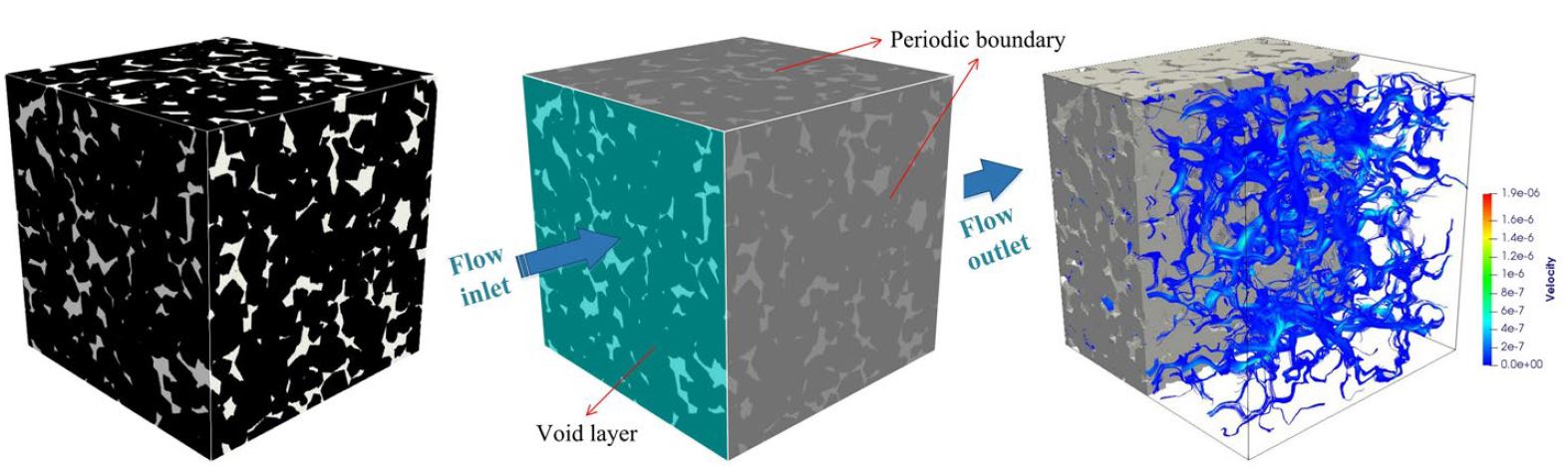 |
||
 |
 |

 |
 |
|
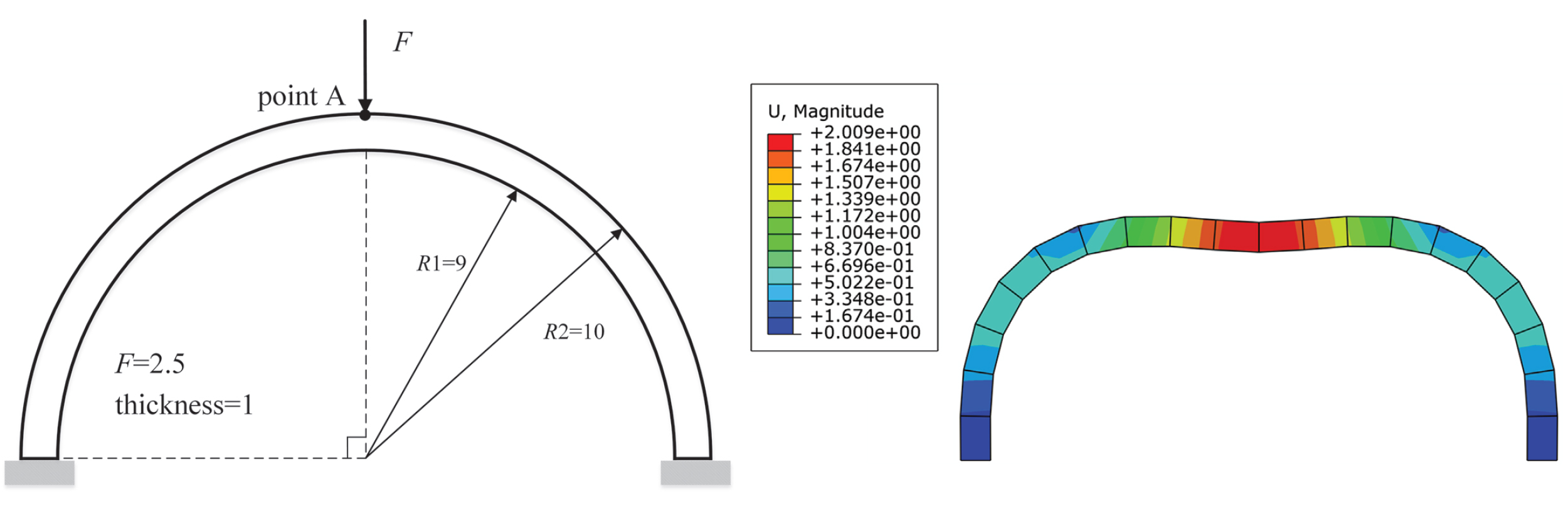 |
||
 |
 |

 |
 |
|
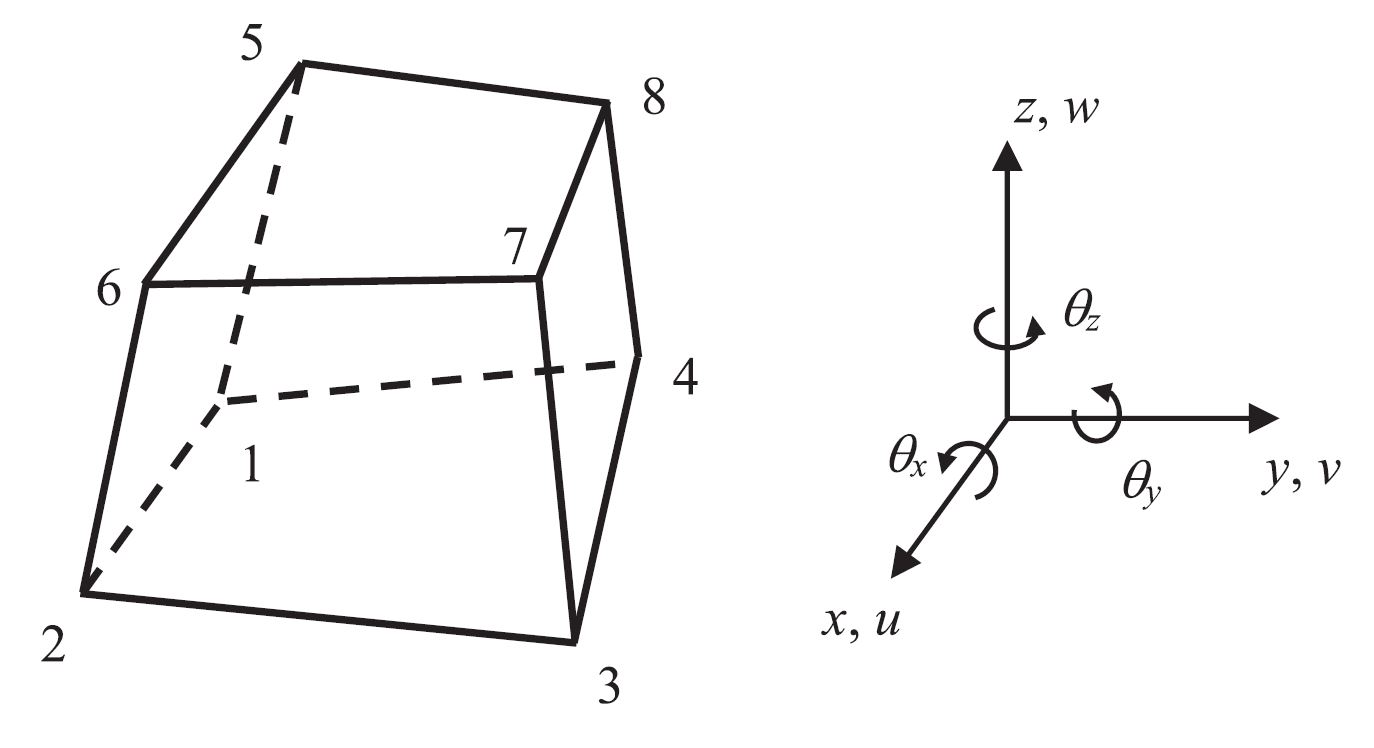 |
||
 |
 |

 |
 |
|
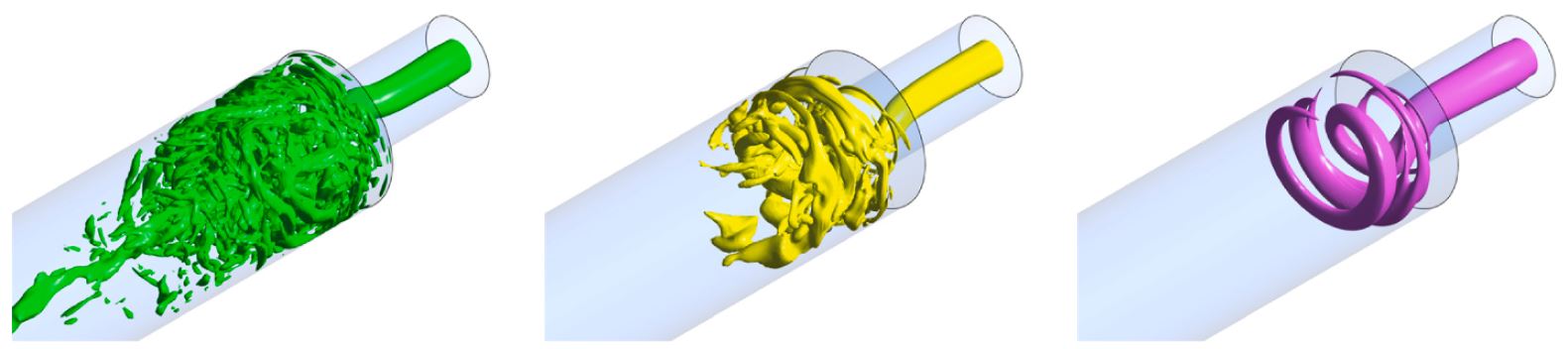 |
||
 |
 |

 |
 |
|
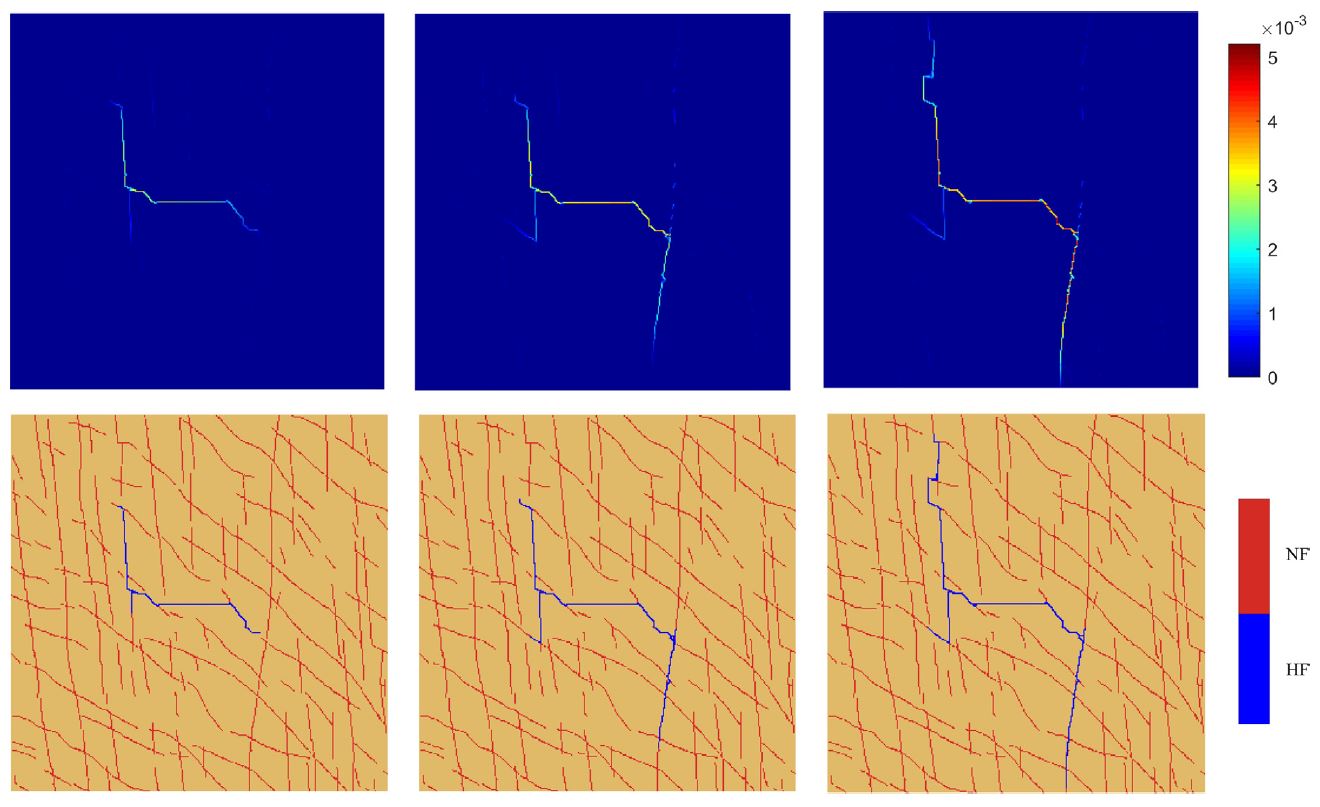 |
||
 |
 |

 |
 |
|
 |
||
 |
 |


2019
 |
 |
|
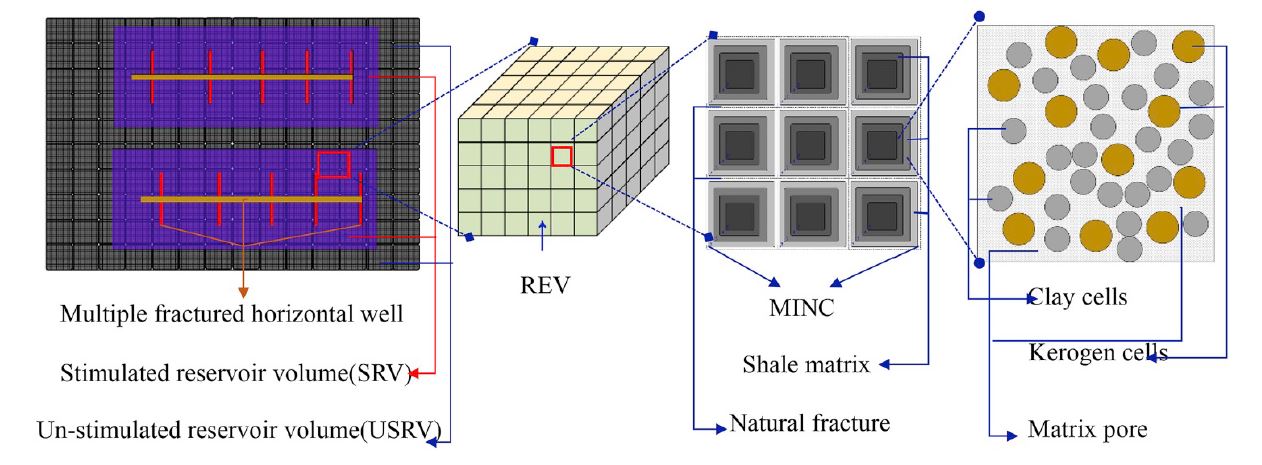 |
||
 |
 |

 |
 |
|
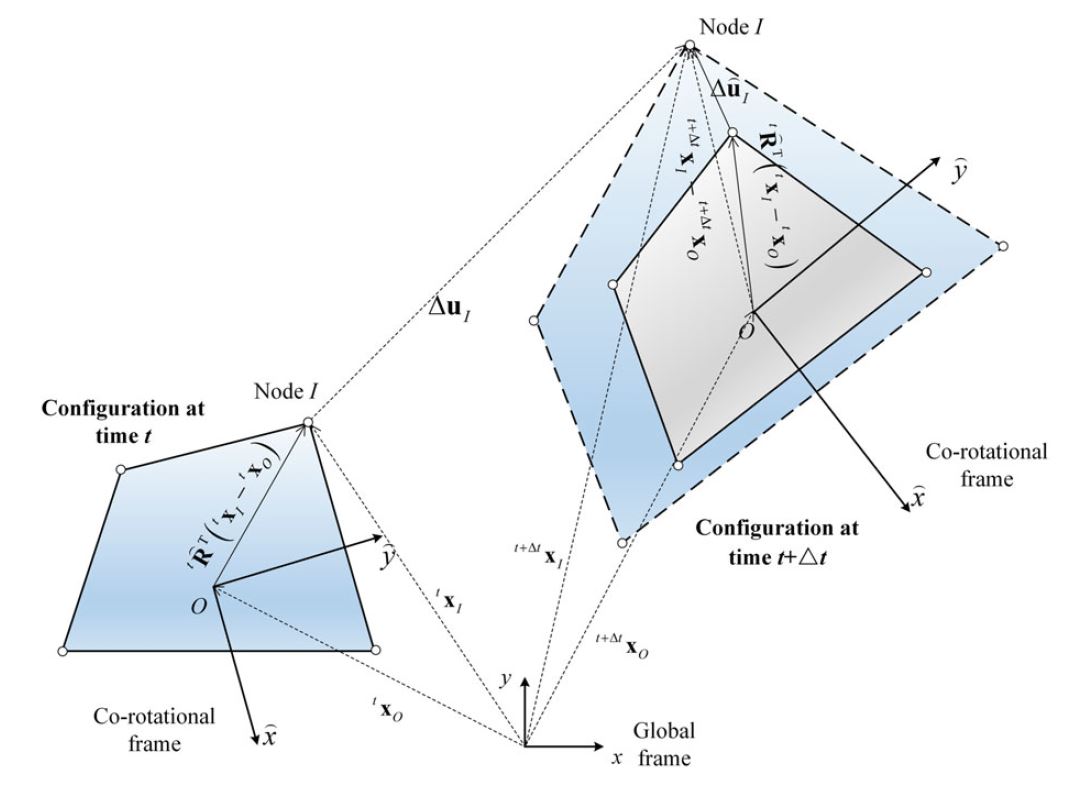 |
||
 |
 |

 |
 |
|
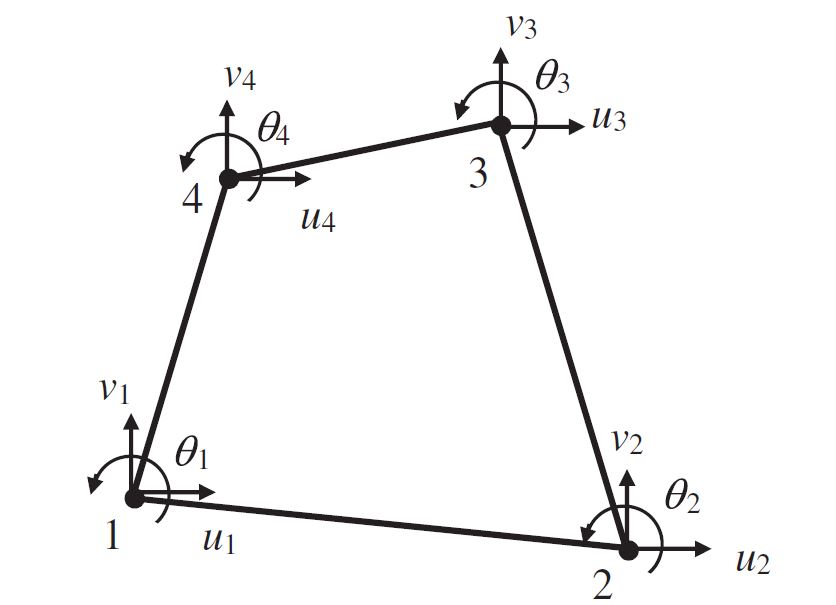 |
||
 |
 |

 |
 |
|
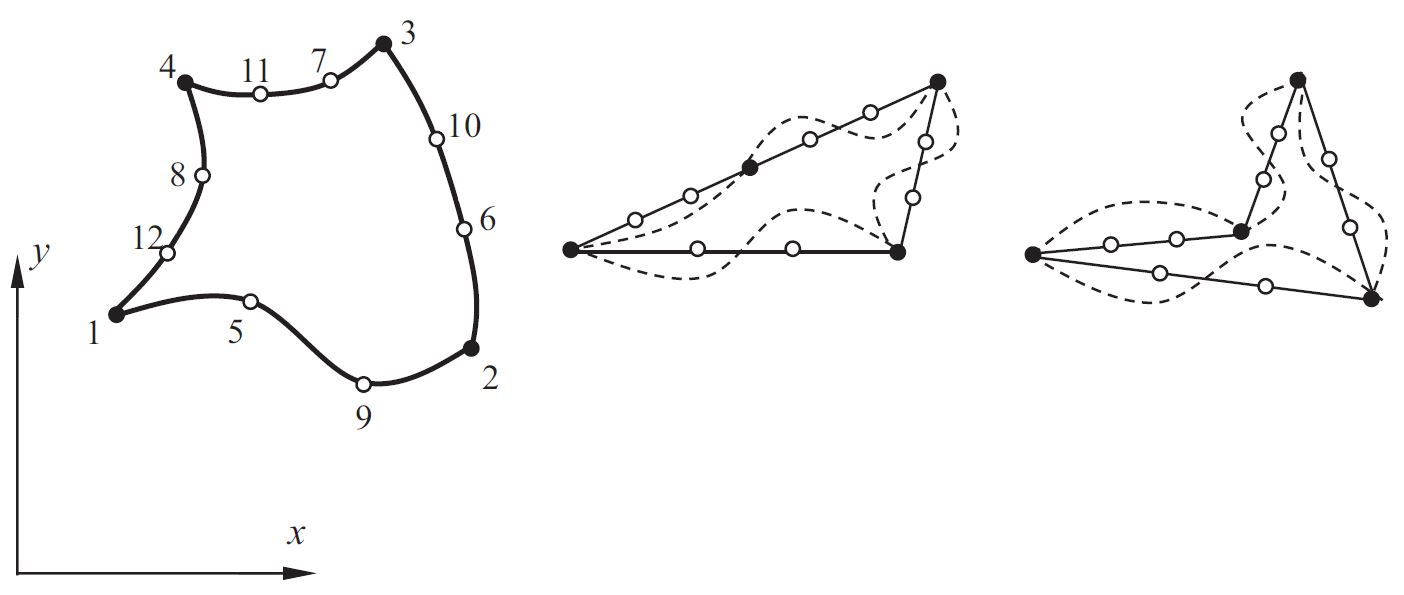 |
||
 |
 |

 |
 |
|
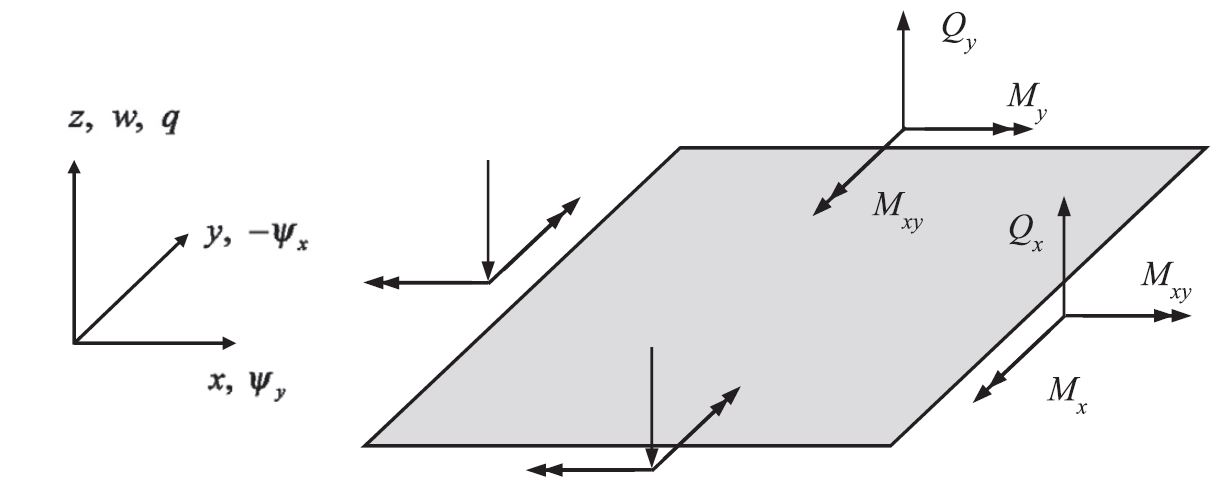 |
||
 |
 |

 |
 |
|
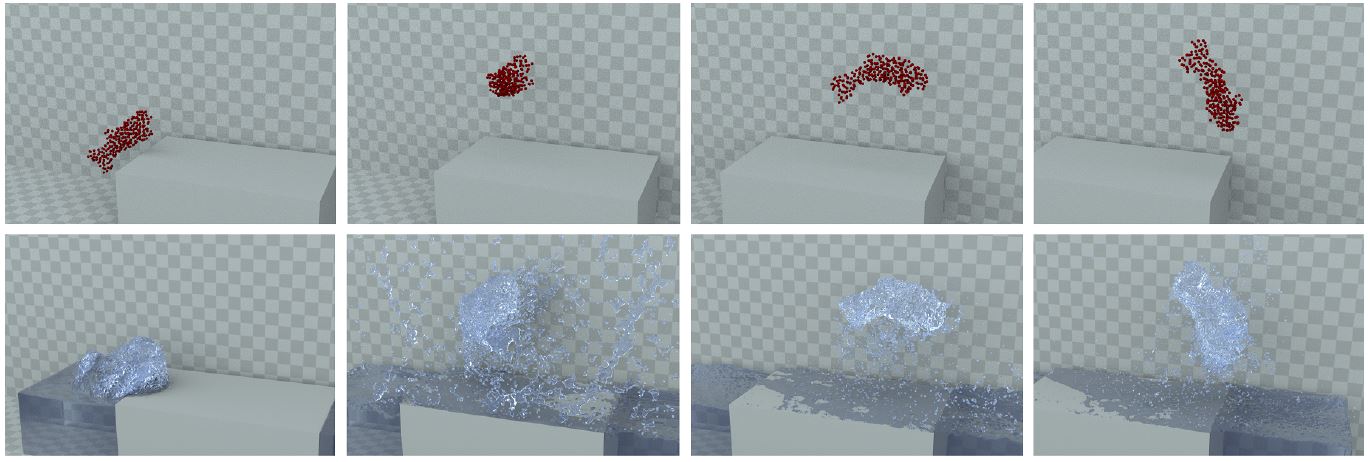 |
||
 |
 |


 |
 |
|
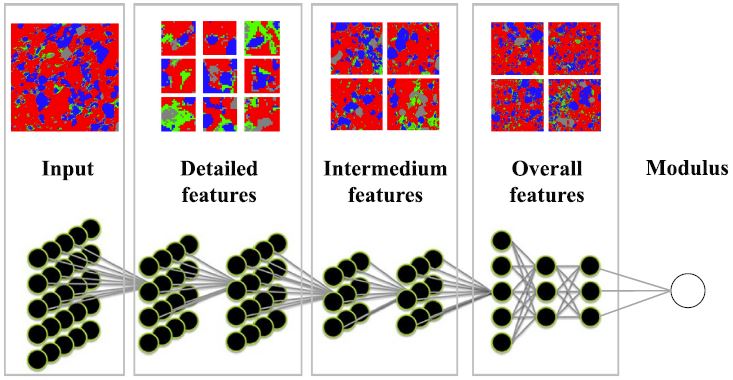 |
||
 |
 |

 |
 |
|
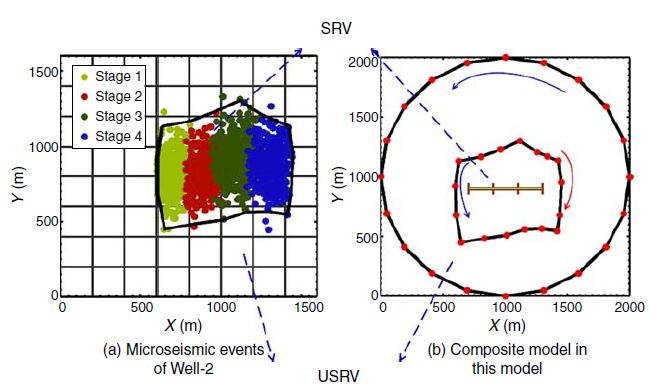 |
||
 |
 |

2018
 |
 |
|
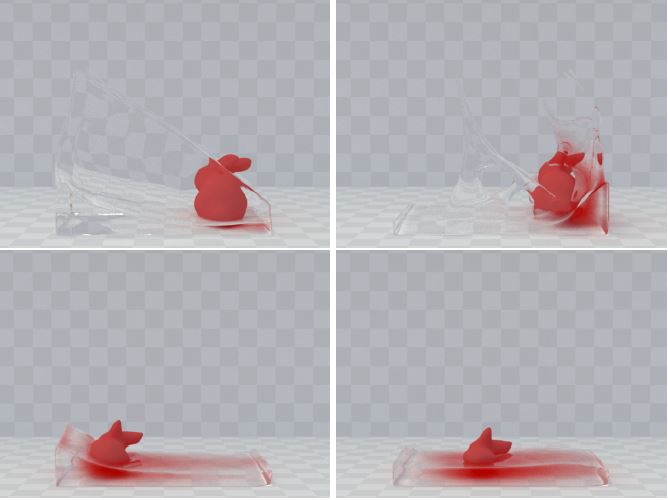 |
||
 |
 |


 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
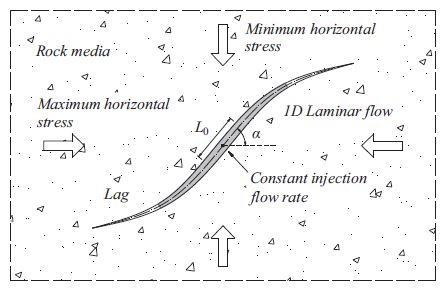 |
||
 |
 |

 |
 |
|
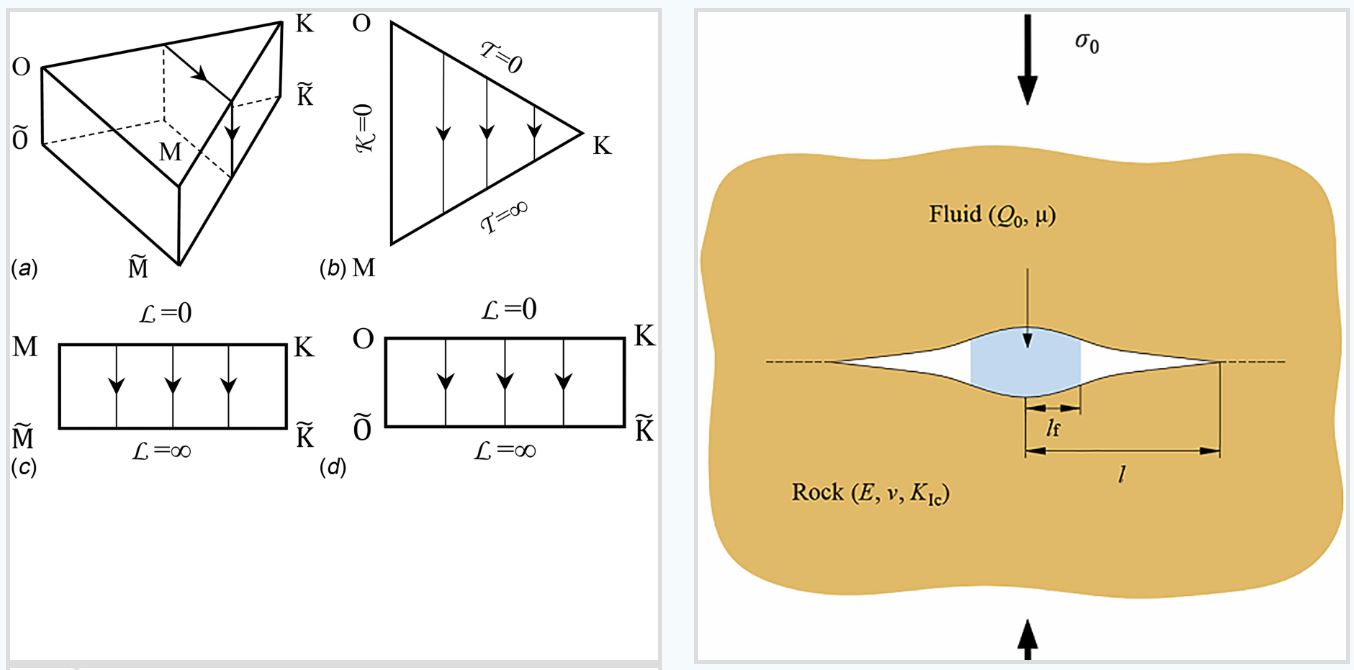 |
||
 |
 |

 |
 |
|
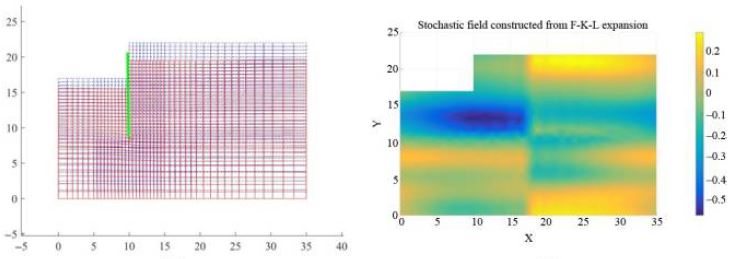 |
||
 |
 |

 |
 |
|
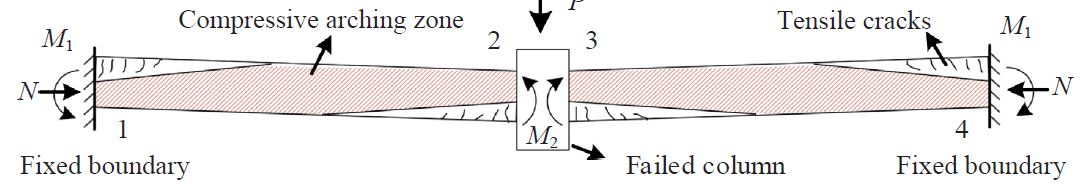 |
||
 |
 |

 |
 |
|
 |
||
 |
 |


2017
 |
 |
|
_element_scheme.jpg) |
||
 |
 |

 |
 |
|
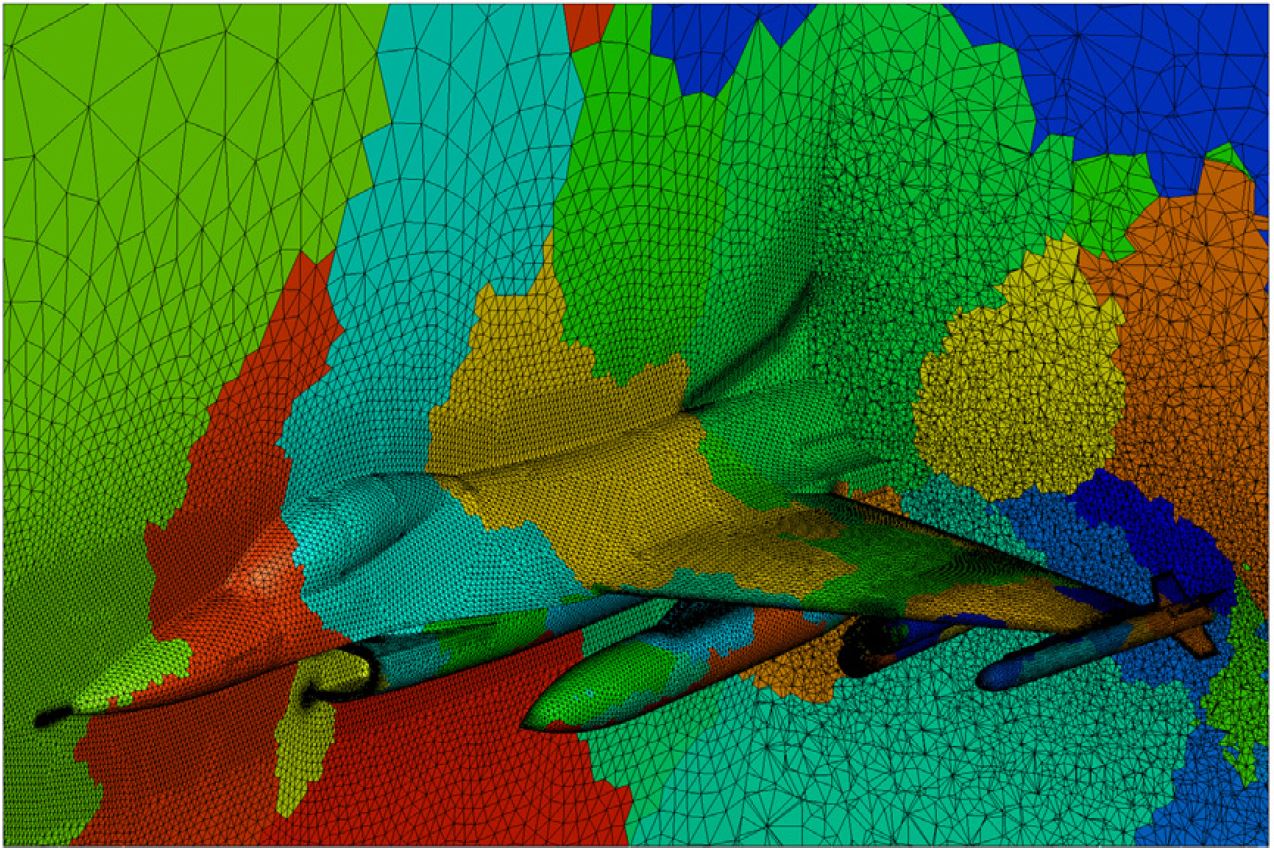 |
||
 |
 |

 |
 |
|
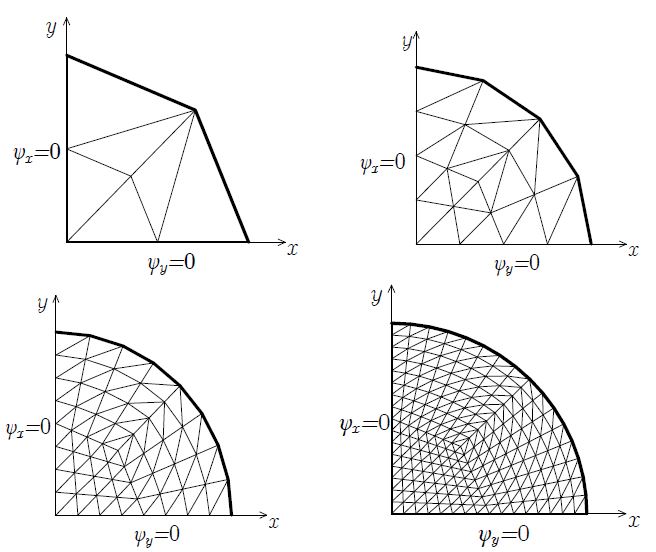 |
||
 |
 |

 |
 |
|
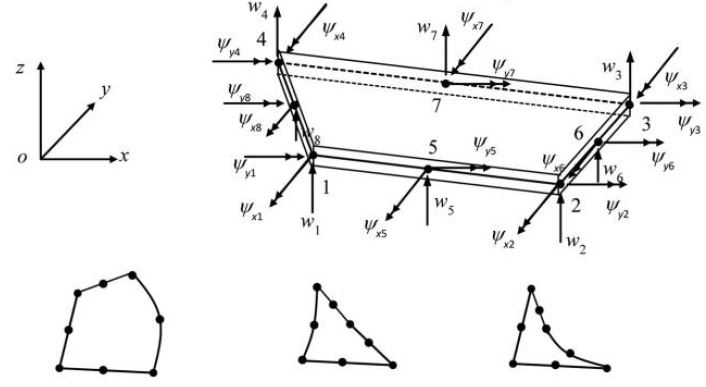 |
||
 |
 |

 |
 |
|
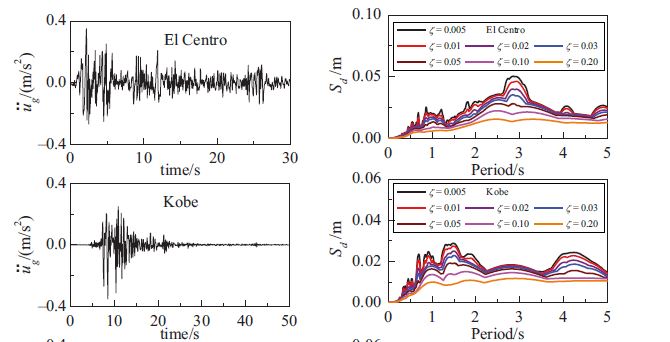 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

2016
 |
 |
|
 |
||
 |
 |


 |
 |
|
 |
||
 |
 |

 |
 |
|
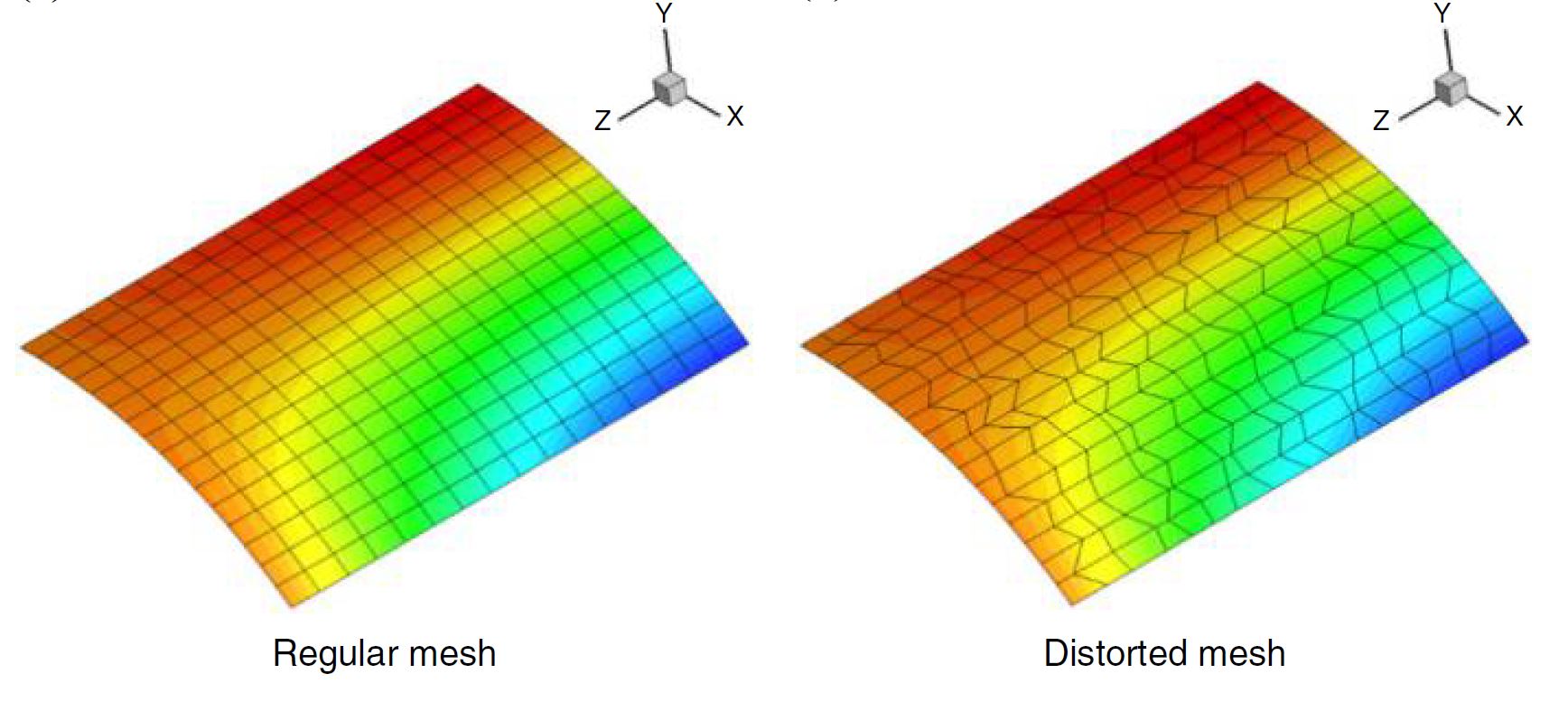 |
||
 |
 |

 |
 |
|
 |
||
 |
 |


 |
 |
|
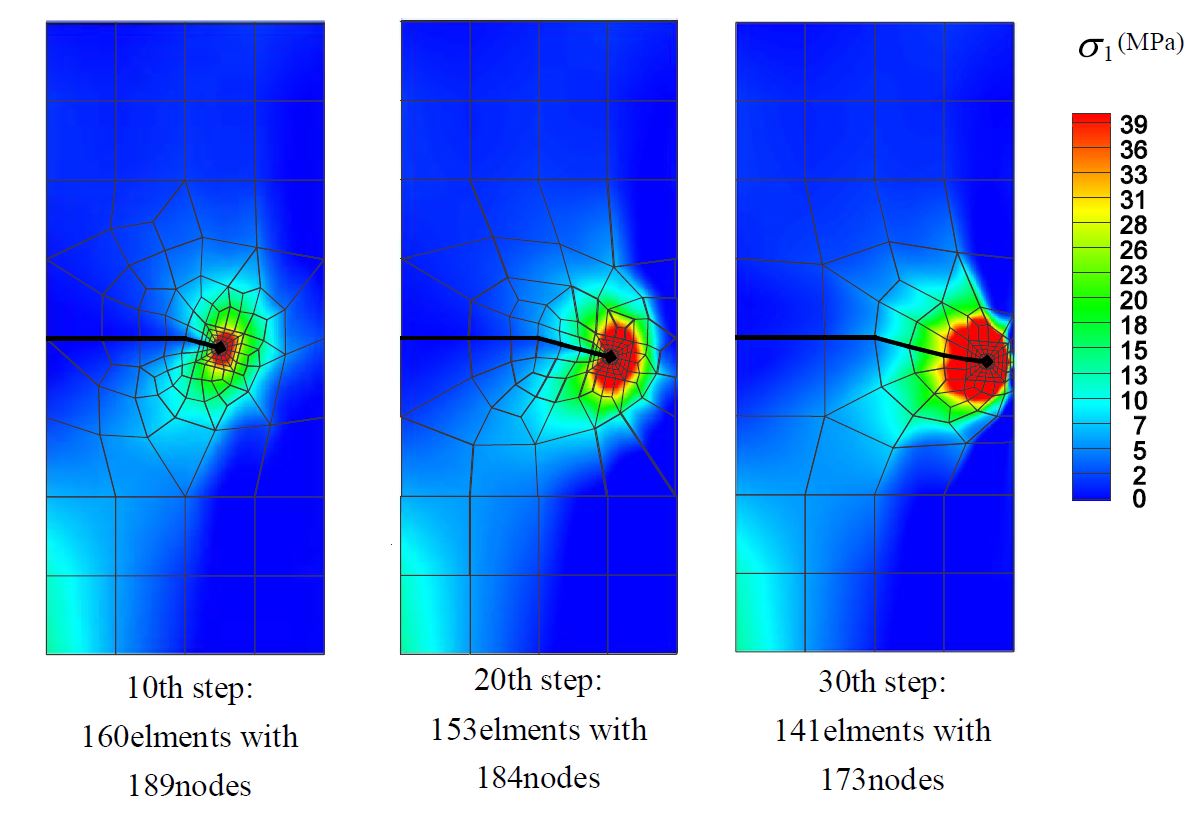 |
||
 |
 |

2015
 |
 |
|
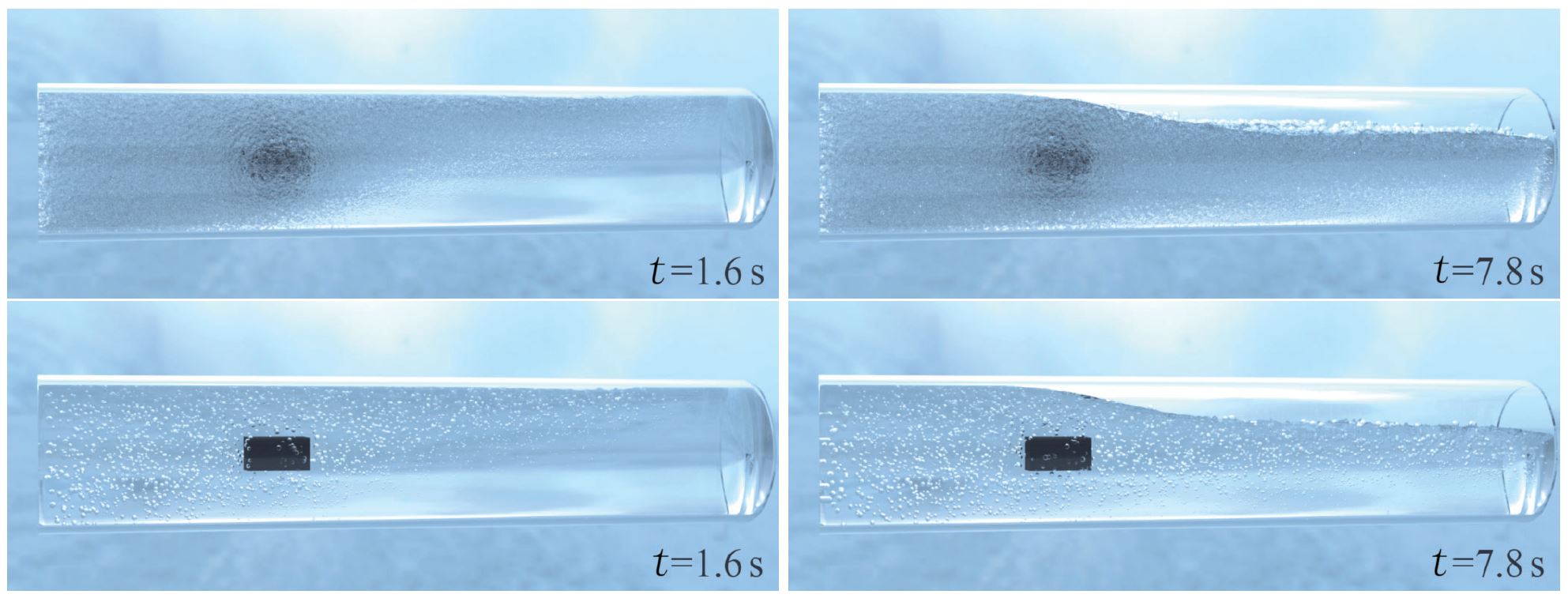 |
||
 |
 |


 |
 |
|
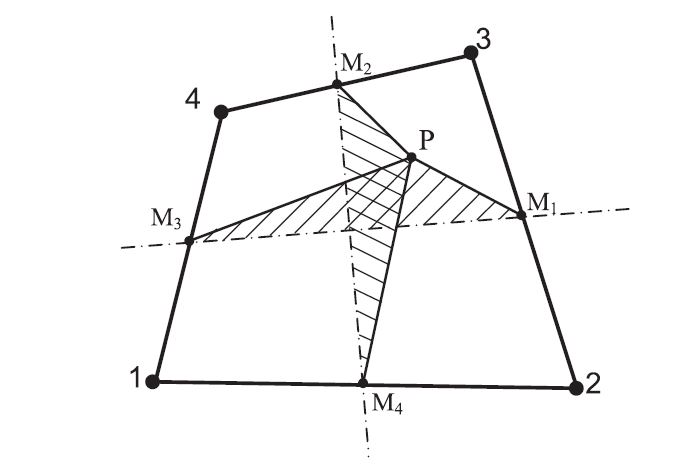 |
||
 |
 |

 |
 |
|
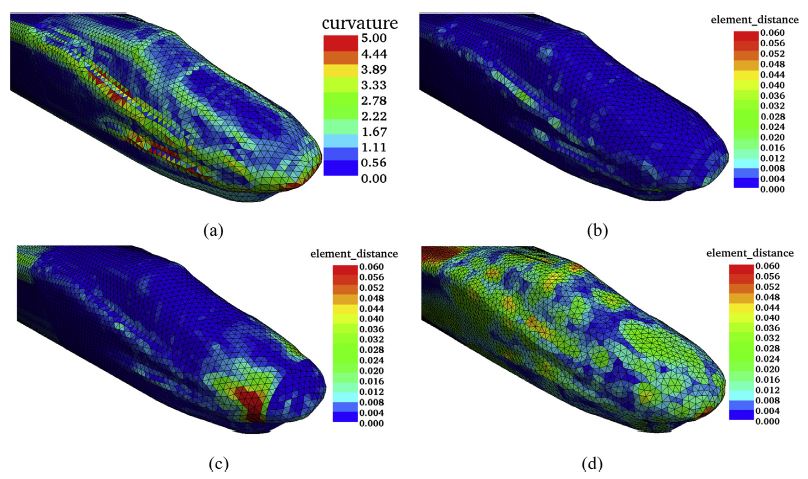 |
||
 |
 |

 |
 |
|
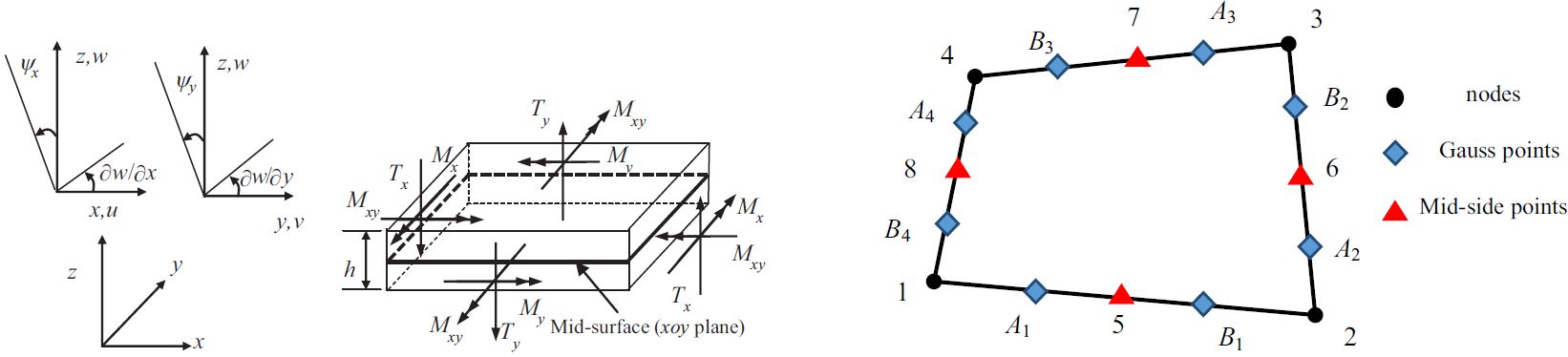 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
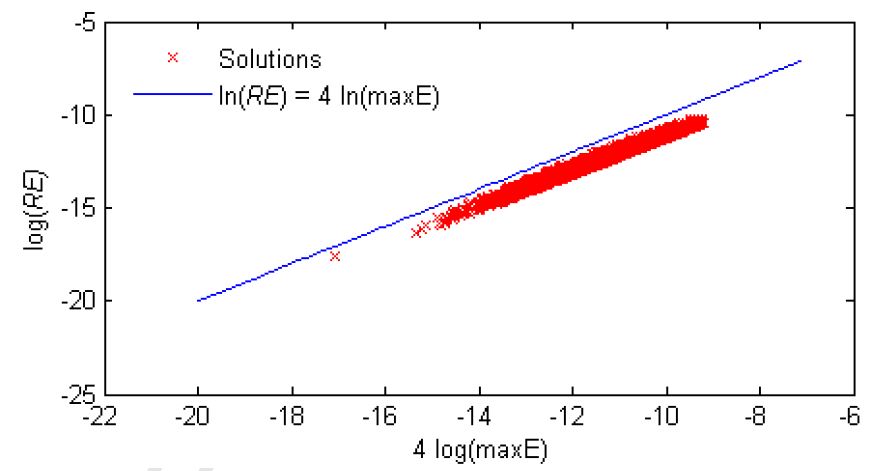 |
||
 |
 |

2014
 |
 |
|
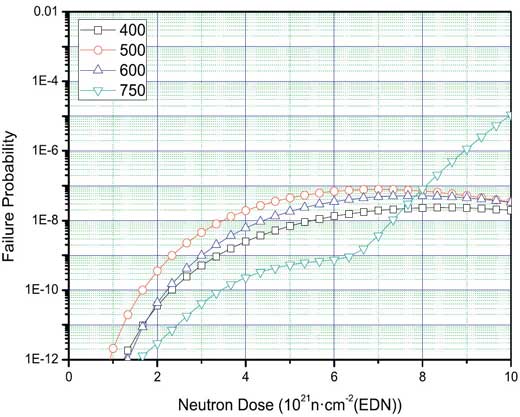 |
||
 |
 |

 |
 |
|
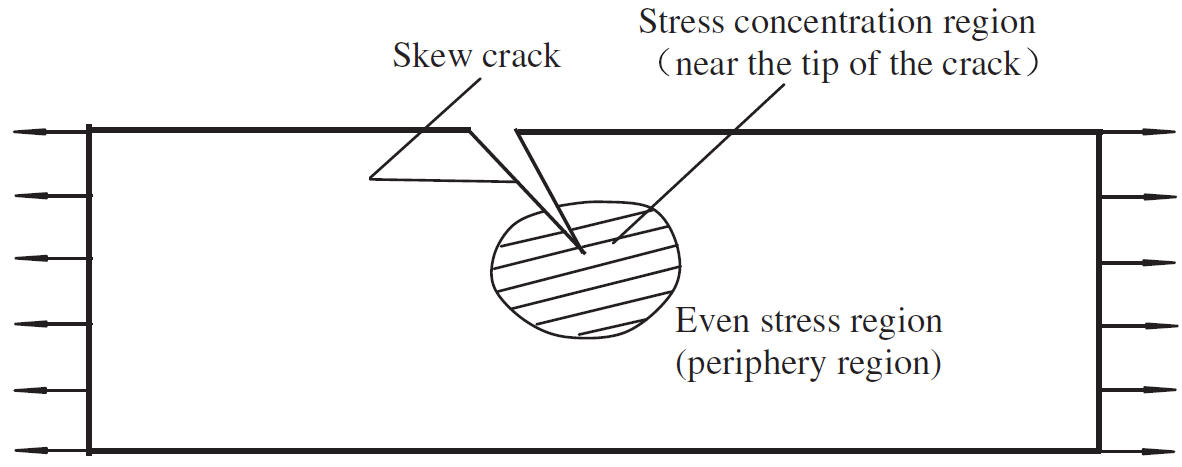 |
||
 |
 |

 |
 |
|
 |
||
 |
 |


 |
 |
|
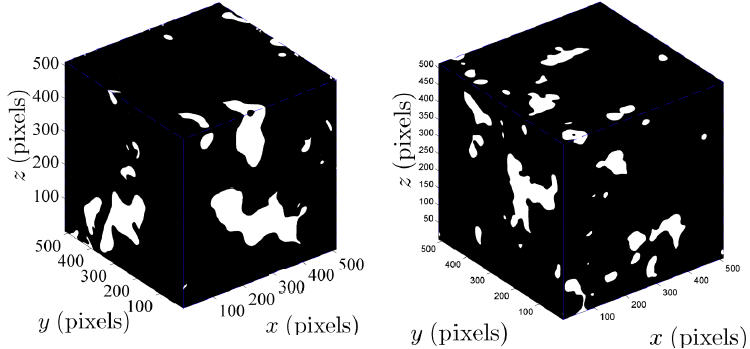 |
||
 |
 |

 |
 |
|
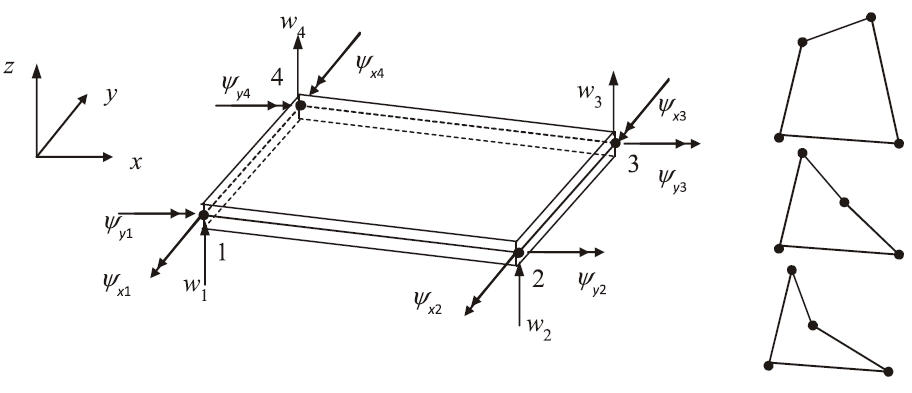 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

2013
 |
 |
|
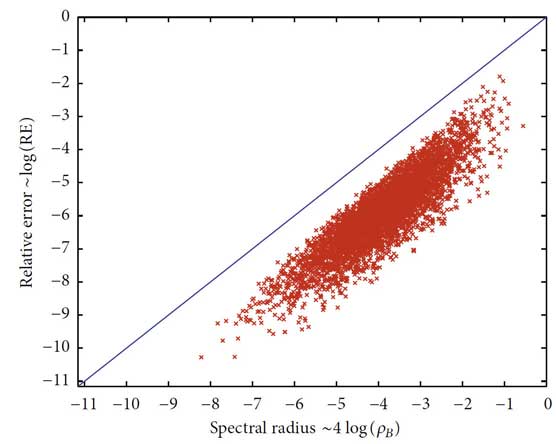 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
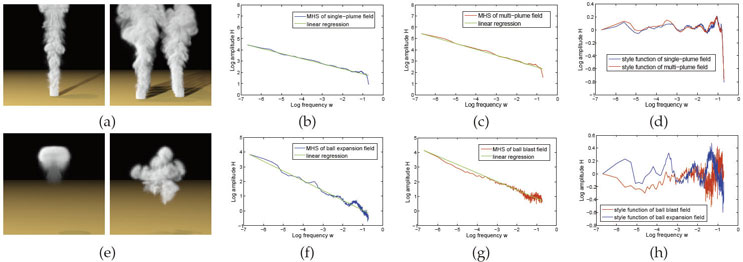 |
||
 |
 |


 |
 |
|
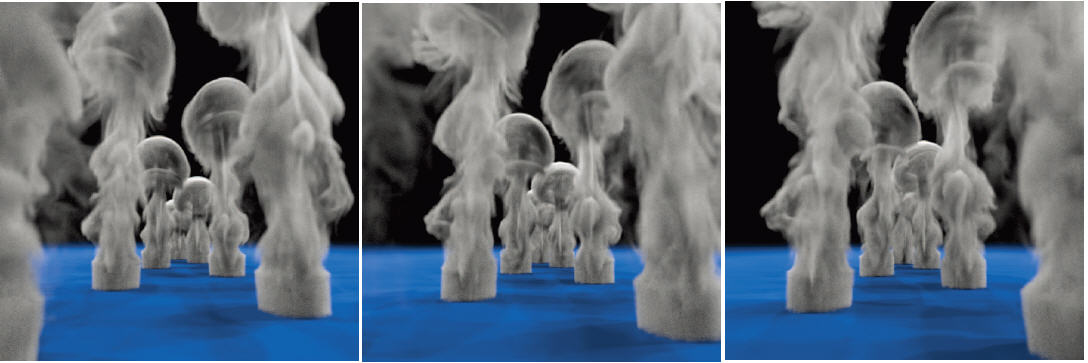 |
||
 |
 |


2012
 |
 |
|
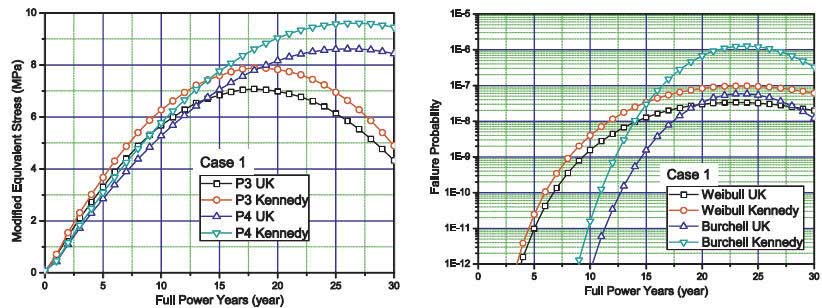 |
||
 |
 |

 |
 |
|
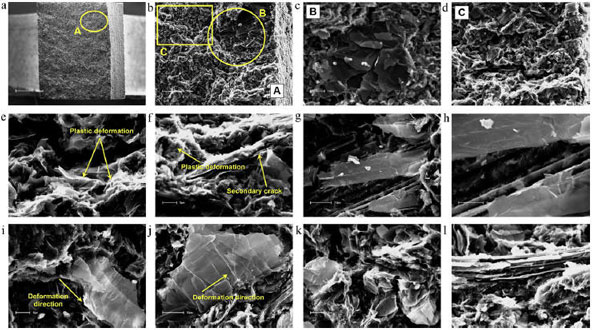 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |


2011
 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

2010
 |
 |
|
 |
||
 |
 |

 |
 |
|
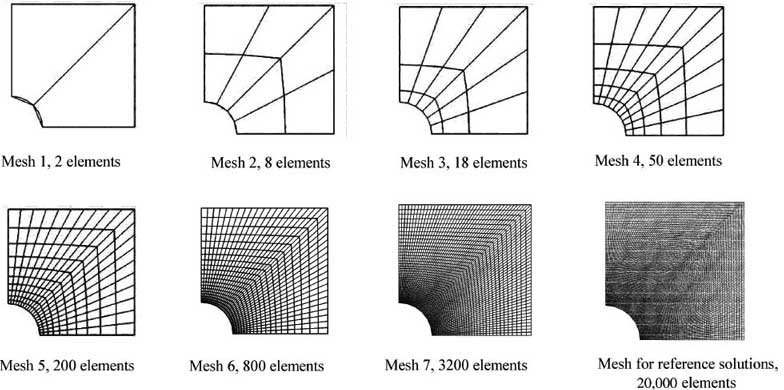 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
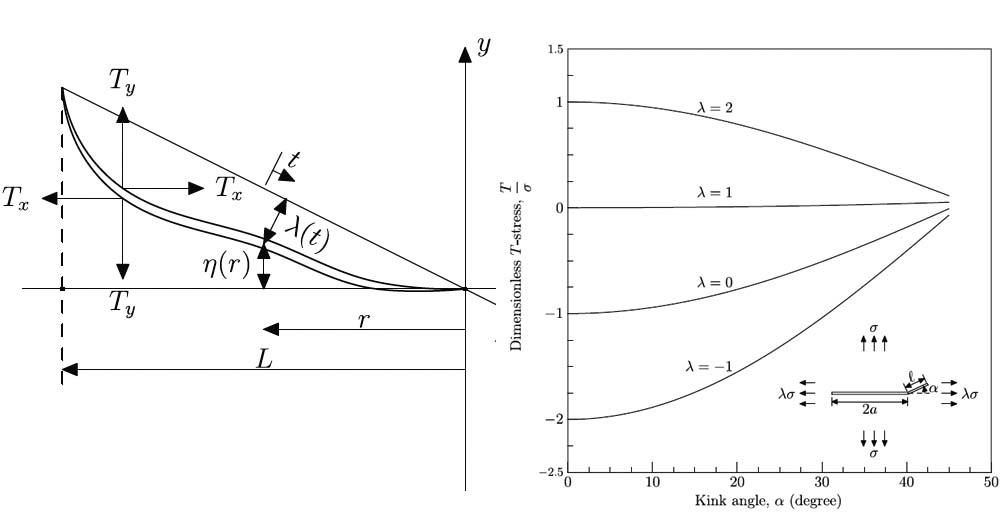 |
||
 |
 |

 |
 |
|
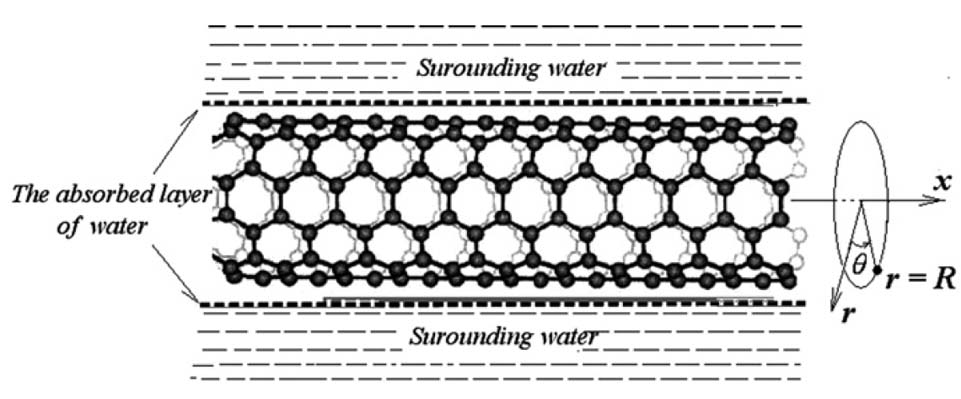 |
||
 |
 |

 |
 |
|
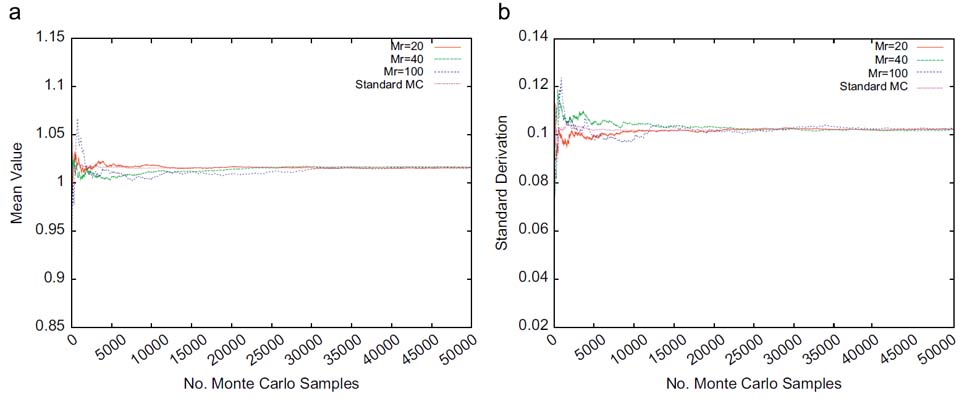 |
||
 |
 |

 |
 |
|
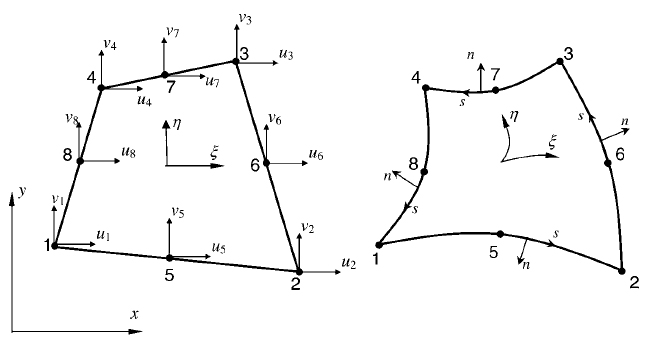 |
||
 |
 |

2009
 |
 |
|
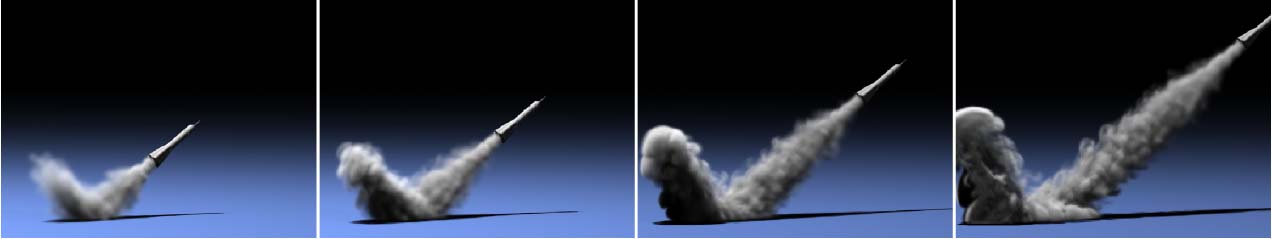 |
||
 |
 |


 |
 |
|
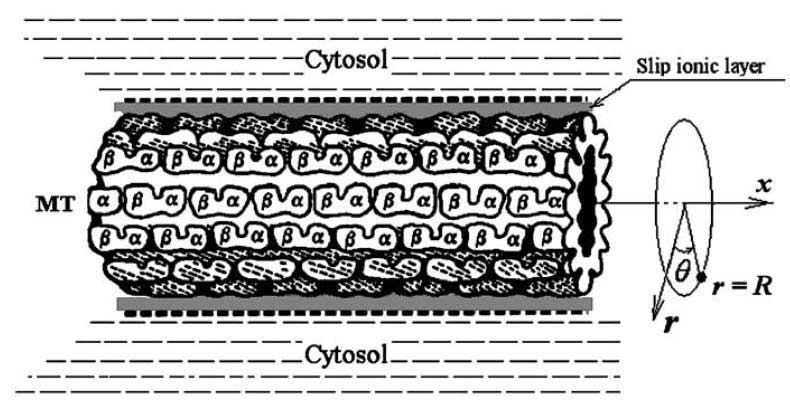 |
||
 |
 |

 |
 |
|
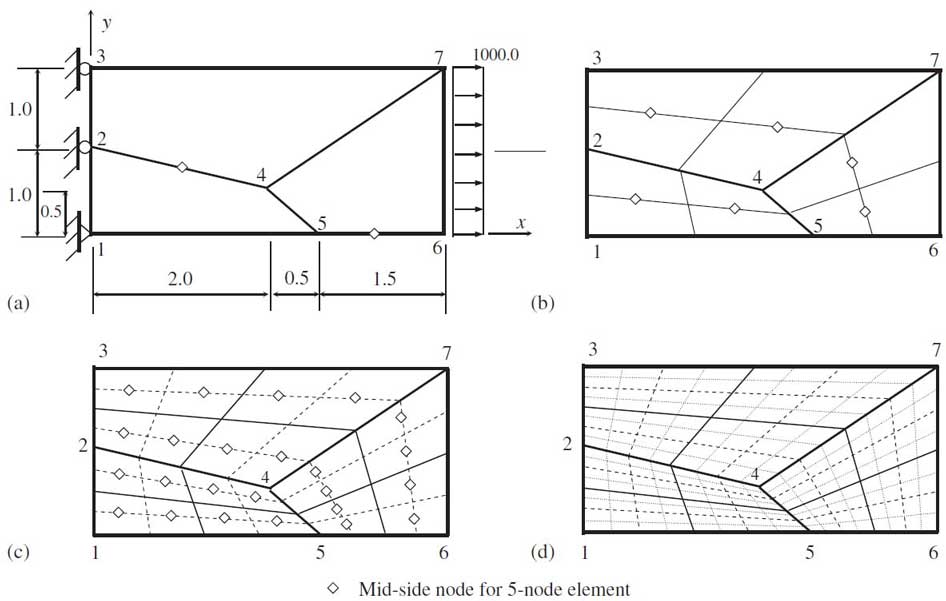 |
||
 |
 |

 |
 |
|
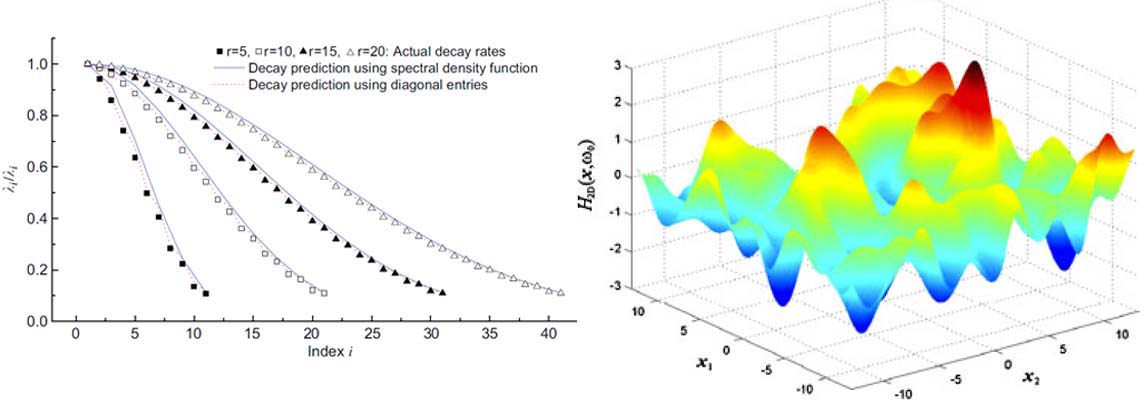 |
||
 |
 |

2008
 |
 |
|
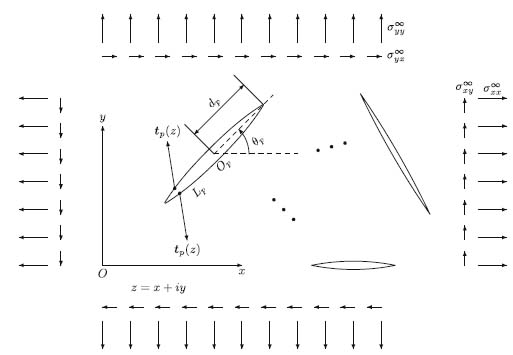 |
||
 |
 |

 |
 |
|
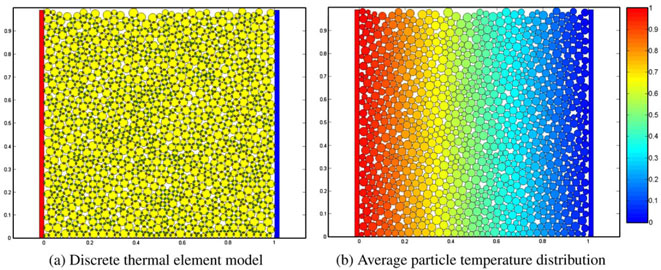 |
||
 |
 |

2006
 |
 |
|
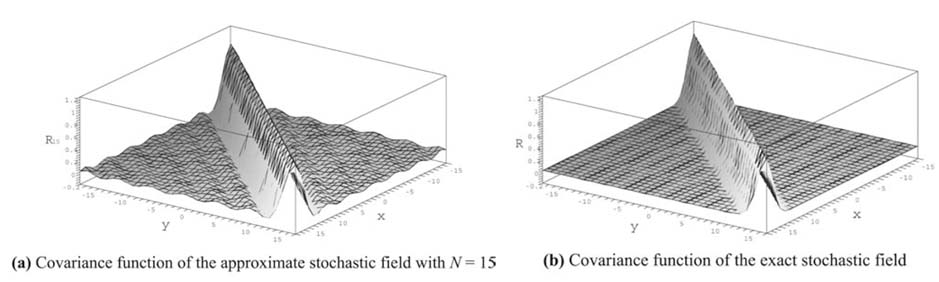 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

 |
 |
|
 |
||
 |
 |

2004
 |
 |
|
 |
||
 |
 |


2002
 |
 |
|
 |
||
 |
 |

 |
 |
|
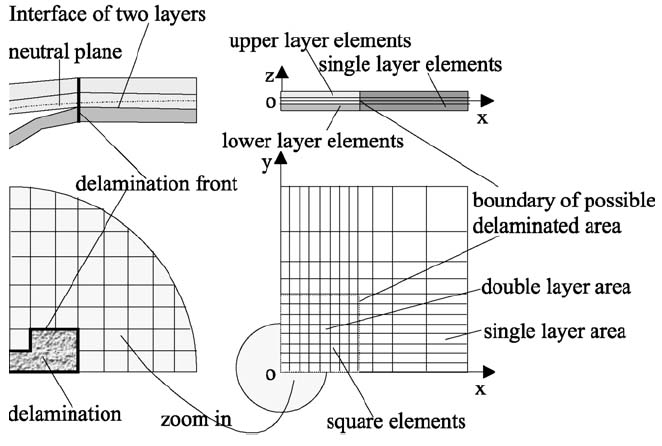 |
||
 |
 |
