Research

Broadly I am interested in control of civil structures under seismic excitation, it includes control of long span bridges (Cable stayed or Suspension bridges) , tall slender buildings, long cables etc. This interest I took up while working with my research supervisor Prof. Ananth Ramaswamy of Department of Civil Engineering, IISc.

While working in base isolated structural control I came in contact with Prof. Anil Kumar Agrawal of CCNY, New York. He has involved me into a new and emerging field of semi-active structural control using Magneto-rheological dampers. It is his help (provided the MR dampers) and my supervisors initiation we started structural dynamics and real time control experiments in structural dynamics laboratory of Department of Civil Engineering, IISc.

My research interest in control of infinite dimensional system (systems governed by PDEs) has bloomed while working with Dr. Radhakant Padhi of Aerospace Engineering Department, IISc.

----------------------------------------------------------------------------------------------------------------------------------------------------

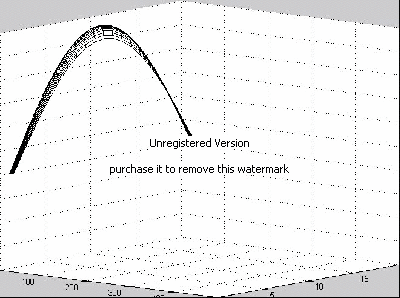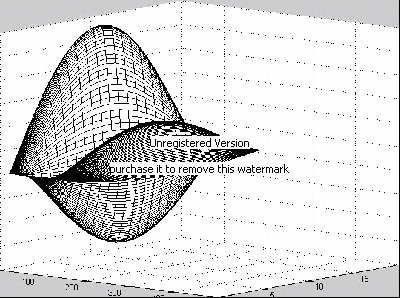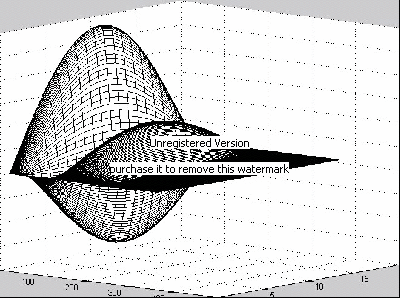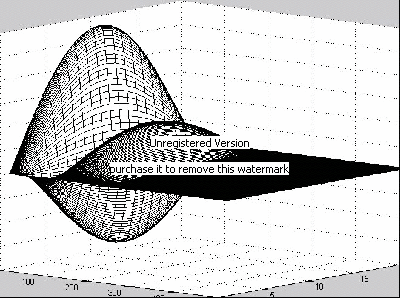[Simply supported beam with control  using optimal dynamic inversion]

Control of Distributed Parameter Systems

Most of Structural elements, like, beams, cables, plates and shells are distributed parameters systems and are governed by partial differential equation (pde) of motion. Discretization of pde’s to finite number of ordinary differential equation’s (odes) brings in with it error due to un-modeled dynamics, especially for nonlinear systems. When controller is designed based on the discretized model, inevitable errors like controller spillover and observer spillover occur. Moreover, for nonlinear systems discretization proves to be fatal. One way to minimize these errors is to design the controller directly based on the pde of the dynamical system. Optimal dynamic inversion is one such technique which guides the structural vibration to the desired trajectory in an optimal way. In this method, dynamic inversion is coupled with optimization theory to obtain a closed form solution for controlling distributed parameter systems. The method is versatile in the sense that it can be applied to both linear and nonlinear systems and can be used to monitor both continuous and discrete actuators.